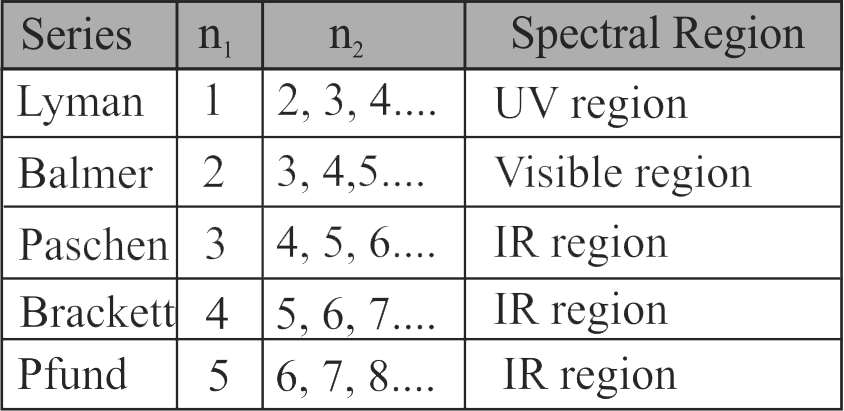
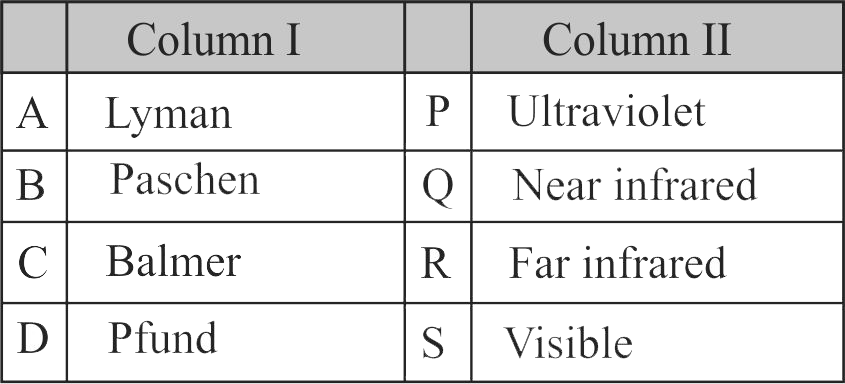
307570 The frequency of radiation emitted when the electron falls from \({\rm{n = 4}}\) to \({\rm{n = 1}}\) in a hydrogen atom will be (Given: ionization energy of \({\rm{H = 2}}{\rm{.18 \times 1}}{{\rm{0}}^{{\rm{ - 18}}}}{\rm{J}}\;{\rm{ato}}{{\rm{m}}^{{\rm{ - 1}}}}\) and \({\rm{h = 6}}{\rm{.625 \times 1}}{{\rm{0}}^{{\rm{ - 34}}}}{\rm{Js)}}\)
307570 The frequency of radiation emitted when the electron falls from \({\rm{n = 4}}\) to \({\rm{n = 1}}\) in a hydrogen atom will be (Given: ionization energy of \({\rm{H = 2}}{\rm{.18 \times 1}}{{\rm{0}}^{{\rm{ - 18}}}}{\rm{J}}\;{\rm{ato}}{{\rm{m}}^{{\rm{ - 1}}}}\) and \({\rm{h = 6}}{\rm{.625 \times 1}}{{\rm{0}}^{{\rm{ - 34}}}}{\rm{Js)}}\)
307570 The frequency of radiation emitted when the electron falls from \({\rm{n = 4}}\) to \({\rm{n = 1}}\) in a hydrogen atom will be (Given: ionization energy of \({\rm{H = 2}}{\rm{.18 \times 1}}{{\rm{0}}^{{\rm{ - 18}}}}{\rm{J}}\;{\rm{ato}}{{\rm{m}}^{{\rm{ - 1}}}}\) and \({\rm{h = 6}}{\rm{.625 \times 1}}{{\rm{0}}^{{\rm{ - 34}}}}{\rm{Js)}}\)
307570 The frequency of radiation emitted when the electron falls from \({\rm{n = 4}}\) to \({\rm{n = 1}}\) in a hydrogen atom will be (Given: ionization energy of \({\rm{H = 2}}{\rm{.18 \times 1}}{{\rm{0}}^{{\rm{ - 18}}}}{\rm{J}}\;{\rm{ato}}{{\rm{m}}^{{\rm{ - 1}}}}\) and \({\rm{h = 6}}{\rm{.625 \times 1}}{{\rm{0}}^{{\rm{ - 34}}}}{\rm{Js)}}\)