358117 Charge \(Q\) is distributed to two different metallic spheres having radii \(R\) and \(2\,R\) such that both spheres have equal surface charge density, then charge on large sphere is

358118 If two charges \(q_{1}\) and \(q_{2}\) are separated by distance
'\(d\)' and placed in a medium of dielectric constant \(k\). What will be the equivalent distance between charges in air for the same electrostatic force?

358119 A small ball of mass \({10^{ - 3}}\;kg\) having a charge of \(1\,\mu C\) is suspended by a string of length \(1.0\;m\). Another identical ball having the same charge is kept at the point of suspension. The minimum horizontal velocity which should be imparted to the lower ball, so that it can make complete revolution is____ \(m/s\). (Take \(\sqrt{10}=3.16\) )

358120 Two identical small equally charged conducting balls are suspended from long threads secured at one point. The charges and masses of the balls are such that they are in equilibrium when the distance between them is \({a}\) (the length of thread \(L > > a\) ). One of the balls is then discharged. Again for the certain value of distance \({b(b < < l)}\) between the balls the equilibrium is restored, find the value of \({\dfrac{a^{3}}{b^{3}}}\) is

358117 Charge \(Q\) is distributed to two different metallic spheres having radii \(R\) and \(2\,R\) such that both spheres have equal surface charge density, then charge on large sphere is

358118 If two charges \(q_{1}\) and \(q_{2}\) are separated by distance
'\(d\)' and placed in a medium of dielectric constant \(k\). What will be the equivalent distance between charges in air for the same electrostatic force?

358119 A small ball of mass \({10^{ - 3}}\;kg\) having a charge of \(1\,\mu C\) is suspended by a string of length \(1.0\;m\). Another identical ball having the same charge is kept at the point of suspension. The minimum horizontal velocity which should be imparted to the lower ball, so that it can make complete revolution is____ \(m/s\). (Take \(\sqrt{10}=3.16\) )

358120 Two identical small equally charged conducting balls are suspended from long threads secured at one point. The charges and masses of the balls are such that they are in equilibrium when the distance between them is \({a}\) (the length of thread \(L > > a\) ). One of the balls is then discharged. Again for the certain value of distance \({b(b < < l)}\) between the balls the equilibrium is restored, find the value of \({\dfrac{a^{3}}{b^{3}}}\) is

358117 Charge \(Q\) is distributed to two different metallic spheres having radii \(R\) and \(2\,R\) such that both spheres have equal surface charge density, then charge on large sphere is

358118 If two charges \(q_{1}\) and \(q_{2}\) are separated by distance
'\(d\)' and placed in a medium of dielectric constant \(k\). What will be the equivalent distance between charges in air for the same electrostatic force?

358119 A small ball of mass \({10^{ - 3}}\;kg\) having a charge of \(1\,\mu C\) is suspended by a string of length \(1.0\;m\). Another identical ball having the same charge is kept at the point of suspension. The minimum horizontal velocity which should be imparted to the lower ball, so that it can make complete revolution is____ \(m/s\). (Take \(\sqrt{10}=3.16\) )

358120 Two identical small equally charged conducting balls are suspended from long threads secured at one point. The charges and masses of the balls are such that they are in equilibrium when the distance between them is \({a}\) (the length of thread \(L > > a\) ). One of the balls is then discharged. Again for the certain value of distance \({b(b < < l)}\) between the balls the equilibrium is restored, find the value of \({\dfrac{a^{3}}{b^{3}}}\) is

358117 Charge \(Q\) is distributed to two different metallic spheres having radii \(R\) and \(2\,R\) such that both spheres have equal surface charge density, then charge on large sphere is

358118 If two charges \(q_{1}\) and \(q_{2}\) are separated by distance
'\(d\)' and placed in a medium of dielectric constant \(k\). What will be the equivalent distance between charges in air for the same electrostatic force?

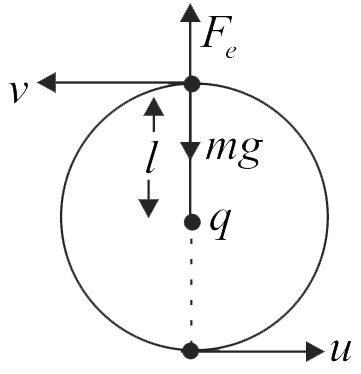
358119 A small ball of mass \({10^{ - 3}}\;kg\) having a charge of \(1\,\mu C\) is suspended by a string of length \(1.0\;m\). Another identical ball having the same charge is kept at the point of suspension. The minimum horizontal velocity which should be imparted to the lower ball, so that it can make complete revolution is____ \(m/s\). (Take \(\sqrt{10}=3.16\) )

358120 Two identical small equally charged conducting balls are suspended from long threads secured at one point. The charges and masses of the balls are such that they are in equilibrium when the distance between them is \({a}\) (the length of thread \(L > > a\) ). One of the balls is then discharged. Again for the certain value of distance \({b(b < < l)}\) between the balls the equilibrium is restored, find the value of \({\dfrac{a^{3}}{b^{3}}}\) is