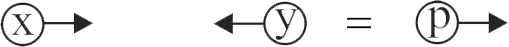357898 A particle \({P}\) is formed due to a completely inelastic collision of particles \({x}\) and \({y}\), having de-Broglie wavelengths \({\lambda_{x}}\) and \({\lambda_{y}}\) respectively. If \({x}\) and \({y}\) were moving in opposite directions, then the de Broglie wavelength of \({p}\) is
357898 A particle \({P}\) is formed due to a completely inelastic collision of particles \({x}\) and \({y}\), having de-Broglie wavelengths \({\lambda_{x}}\) and \({\lambda_{y}}\) respectively. If \({x}\) and \({y}\) were moving in opposite directions, then the de Broglie wavelength of \({p}\) is
357898 A particle \({P}\) is formed due to a completely inelastic collision of particles \({x}\) and \({y}\), having de-Broglie wavelengths \({\lambda_{x}}\) and \({\lambda_{y}}\) respectively. If \({x}\) and \({y}\) were moving in opposite directions, then the de Broglie wavelength of \({p}\) is
357898 A particle \({P}\) is formed due to a completely inelastic collision of particles \({x}\) and \({y}\), having de-Broglie wavelengths \({\lambda_{x}}\) and \({\lambda_{y}}\) respectively. If \({x}\) and \({y}\) were moving in opposite directions, then the de Broglie wavelength of \({p}\) is
357898 A particle \({P}\) is formed due to a completely inelastic collision of particles \({x}\) and \({y}\), having de-Broglie wavelengths \({\lambda_{x}}\) and \({\lambda_{y}}\) respectively. If \({x}\) and \({y}\) were moving in opposite directions, then the de Broglie wavelength of \({p}\) is