357640 A 5 watt source emits monochromatic light of wavelength \(5000\mathop A\limits^o \). When placed \(0.5\;m\) away, it liberates photoelectrons from a photosensitive metallic surface. When the source is moved to a distance of \(2.0\;m\), the number of photoelectrons liberated will be reduced by a factor of
357642
Read the Statement -A and Statement -B carefully to mark the correct options given below:
Statement A :
Figure shows the variation of stopping potential \((V)\) with frequency \((f)\) for the two photo sensitive materials \({M_1}\) and \({M_2}.\) The slope gives value of \(\frac{h}{e}\,\,,\) where \(h\) is Planck’s constant, \(e\) is the charge of electron.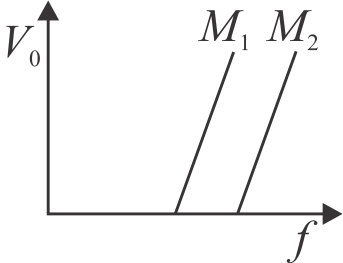
Statement B :
\({M_2}\) will emit photoelectrons of greater kinetic energy for the incident radiation having same frequency.
357643
For intensity \(I\) of a light of wavelength \(6000\)\( \mathop A^{~~\circ} \), the photoelectron saturation current is \(0.40 \,\mu {A}\) and stopping potential is \(1.37 {~V}\). The work function of metal is
(Take \({h}=4.14 \times 10^{-15} {eVs}\) )
357640 A 5 watt source emits monochromatic light of wavelength \(5000\mathop A\limits^o \). When placed \(0.5\;m\) away, it liberates photoelectrons from a photosensitive metallic surface. When the source is moved to a distance of \(2.0\;m\), the number of photoelectrons liberated will be reduced by a factor of
357642
Read the Statement -A and Statement -B carefully to mark the correct options given below:
Statement A :
Figure shows the variation of stopping potential \((V)\) with frequency \((f)\) for the two photo sensitive materials \({M_1}\) and \({M_2}.\) The slope gives value of \(\frac{h}{e}\,\,,\) where \(h\) is Planck’s constant, \(e\) is the charge of electron.
Statement B :
\({M_2}\) will emit photoelectrons of greater kinetic energy for the incident radiation having same frequency.
357643
For intensity \(I\) of a light of wavelength \(6000\)\( \mathop A^{~~\circ} \), the photoelectron saturation current is \(0.40 \,\mu {A}\) and stopping potential is \(1.37 {~V}\). The work function of metal is
(Take \({h}=4.14 \times 10^{-15} {eVs}\) )
357640 A 5 watt source emits monochromatic light of wavelength \(5000\mathop A\limits^o \). When placed \(0.5\;m\) away, it liberates photoelectrons from a photosensitive metallic surface. When the source is moved to a distance of \(2.0\;m\), the number of photoelectrons liberated will be reduced by a factor of
357642
Read the Statement -A and Statement -B carefully to mark the correct options given below:
Statement A :
Figure shows the variation of stopping potential \((V)\) with frequency \((f)\) for the two photo sensitive materials \({M_1}\) and \({M_2}.\) The slope gives value of \(\frac{h}{e}\,\,,\) where \(h\) is Planck’s constant, \(e\) is the charge of electron.
Statement B :
\({M_2}\) will emit photoelectrons of greater kinetic energy for the incident radiation having same frequency.
357643
For intensity \(I\) of a light of wavelength \(6000\)\( \mathop A^{~~\circ} \), the photoelectron saturation current is \(0.40 \,\mu {A}\) and stopping potential is \(1.37 {~V}\). The work function of metal is
(Take \({h}=4.14 \times 10^{-15} {eVs}\) )
357640 A 5 watt source emits monochromatic light of wavelength \(5000\mathop A\limits^o \). When placed \(0.5\;m\) away, it liberates photoelectrons from a photosensitive metallic surface. When the source is moved to a distance of \(2.0\;m\), the number of photoelectrons liberated will be reduced by a factor of
357642
Read the Statement -A and Statement -B carefully to mark the correct options given below:
Statement A :
Figure shows the variation of stopping potential \((V)\) with frequency \((f)\) for the two photo sensitive materials \({M_1}\) and \({M_2}.\) The slope gives value of \(\frac{h}{e}\,\,,\) where \(h\) is Planck’s constant, \(e\) is the charge of electron.
Statement B :
\({M_2}\) will emit photoelectrons of greater kinetic energy for the incident radiation having same frequency.
357643
For intensity \(I\) of a light of wavelength \(6000\)\( \mathop A^{~~\circ} \), the photoelectron saturation current is \(0.40 \,\mu {A}\) and stopping potential is \(1.37 {~V}\). The work function of metal is
(Take \({h}=4.14 \times 10^{-15} {eVs}\) )