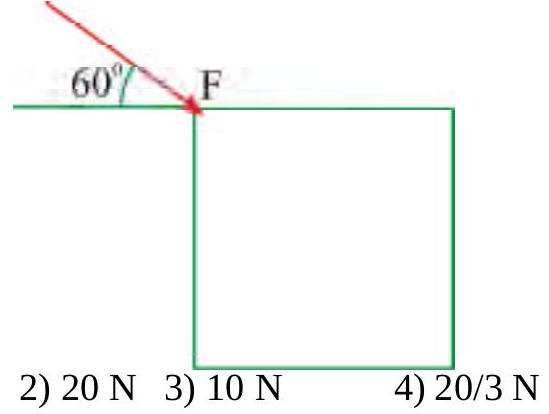
270288 A block weighing\(10 \mathrm{~kg}\) is at rest on a horizontal table. The coefficient of static friction between the block and the table is 0.5. If a force acts downward at \(60^{\circ}\) with the horizontal, how large can it be without causing the block to move? \(\left(g=10 \mathrm{~ms}^{-2}\right)\)
270288 A block weighing\(10 \mathrm{~kg}\) is at rest on a horizontal table. The coefficient of static friction between the block and the table is 0.5. If a force acts downward at \(60^{\circ}\) with the horizontal, how large can it be without causing the block to move? \(\left(g=10 \mathrm{~ms}^{-2}\right)\)
270288 A block weighing\(10 \mathrm{~kg}\) is at rest on a horizontal table. The coefficient of static friction between the block and the table is 0.5. If a force acts downward at \(60^{\circ}\) with the horizontal, how large can it be without causing the block to move? \(\left(g=10 \mathrm{~ms}^{-2}\right)\)
270288 A block weighing\(10 \mathrm{~kg}\) is at rest on a horizontal table. The coefficient of static friction between the block and the table is 0.5. If a force acts downward at \(60^{\circ}\) with the horizontal, how large can it be without causing the block to move? \(\left(g=10 \mathrm{~ms}^{-2}\right)\)
270288 A block weighing\(10 \mathrm{~kg}\) is at rest on a horizontal table. The coefficient of static friction between the block and the table is 0.5. If a force acts downward at \(60^{\circ}\) with the horizontal, how large can it be without causing the block to move? \(\left(g=10 \mathrm{~ms}^{-2}\right)\)