269910 A Person crossing a road with a certain velocity due north, sees a car moving towards east. The relative velocity of the car w.r.t the person is\(\sqrt{2}\) times that of the velocity of the person. The angle made by the relative velocity with the east is

269911 A Person is walking in rain feels the velocity of rain as twice to hisvelocity . At which angle he should hold the umbrella with vertical if he moves forward, if it is raining vertically downwards

269912 When it is raining vertically down, to a man walking on road the velocity of rain appears to be 1.5 times hisvelocity . To protect himself from rain he should hold the umbrella at an angle \(\theta\) to vertical. Then \(\tan \theta=\)

269913 A motor car\(\mathbf{A}\) is travelling with a velocity of \(20 \mathrm{~m} / \mathrm{s}\) in the north - west direction and another motor car B is travelling with a velocity of \(15 \mathrm{~m} / \mathrm{s}\) in the north-east direction. The magnitude of relative velocity of \(B\) with respect to \(A\) is.

269910 A Person crossing a road with a certain velocity due north, sees a car moving towards east. The relative velocity of the car w.r.t the person is\(\sqrt{2}\) times that of the velocity of the person. The angle made by the relative velocity with the east is

269911 A Person is walking in rain feels the velocity of rain as twice to hisvelocity . At which angle he should hold the umbrella with vertical if he moves forward, if it is raining vertically downwards

269912 When it is raining vertically down, to a man walking on road the velocity of rain appears to be 1.5 times hisvelocity . To protect himself from rain he should hold the umbrella at an angle \(\theta\) to vertical. Then \(\tan \theta=\)

269913 A motor car\(\mathbf{A}\) is travelling with a velocity of \(20 \mathrm{~m} / \mathrm{s}\) in the north - west direction and another motor car B is travelling with a velocity of \(15 \mathrm{~m} / \mathrm{s}\) in the north-east direction. The magnitude of relative velocity of \(B\) with respect to \(A\) is.

269910 A Person crossing a road with a certain velocity due north, sees a car moving towards east. The relative velocity of the car w.r.t the person is\(\sqrt{2}\) times that of the velocity of the person. The angle made by the relative velocity with the east is

269911 A Person is walking in rain feels the velocity of rain as twice to hisvelocity . At which angle he should hold the umbrella with vertical if he moves forward, if it is raining vertically downwards

269912 When it is raining vertically down, to a man walking on road the velocity of rain appears to be 1.5 times hisvelocity . To protect himself from rain he should hold the umbrella at an angle \(\theta\) to vertical. Then \(\tan \theta=\)

269913 A motor car\(\mathbf{A}\) is travelling with a velocity of \(20 \mathrm{~m} / \mathrm{s}\) in the north - west direction and another motor car B is travelling with a velocity of \(15 \mathrm{~m} / \mathrm{s}\) in the north-east direction. The magnitude of relative velocity of \(B\) with respect to \(A\) is.

269910 A Person crossing a road with a certain velocity due north, sees a car moving towards east. The relative velocity of the car w.r.t the person is\(\sqrt{2}\) times that of the velocity of the person. The angle made by the relative velocity with the east is

269911 A Person is walking in rain feels the velocity of rain as twice to hisvelocity . At which angle he should hold the umbrella with vertical if he moves forward, if it is raining vertically downwards

269912 When it is raining vertically down, to a man walking on road the velocity of rain appears to be 1.5 times hisvelocity . To protect himself from rain he should hold the umbrella at an angle \(\theta\) to vertical. Then \(\tan \theta=\)

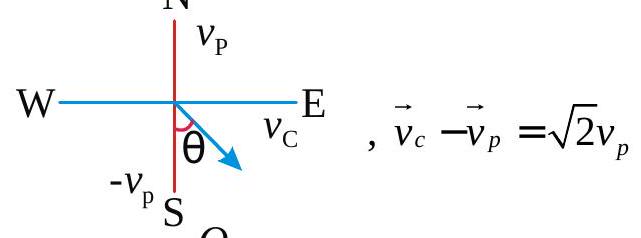
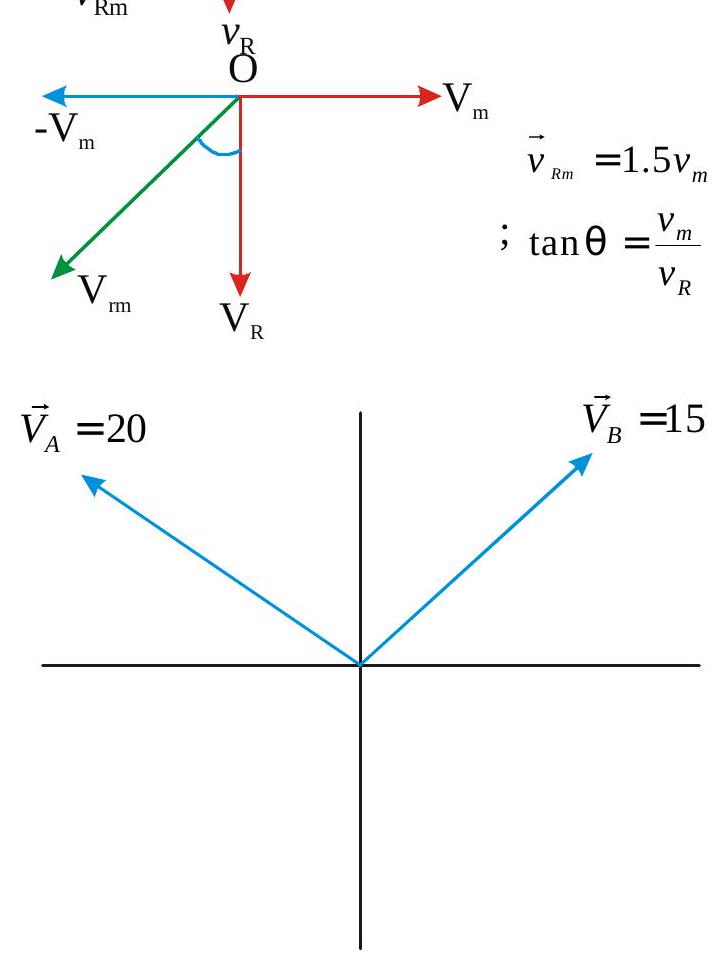
269913 A motor car\(\mathbf{A}\) is travelling with a velocity of \(20 \mathrm{~m} / \mathrm{s}\) in the north - west direction and another motor car B is travelling with a velocity of \(15 \mathrm{~m} / \mathrm{s}\) in the north-east direction. The magnitude of relative velocity of \(B\) with respect to \(A\) is.