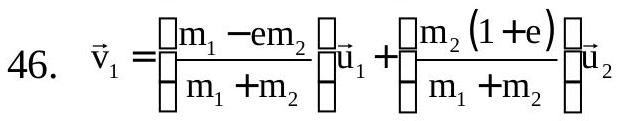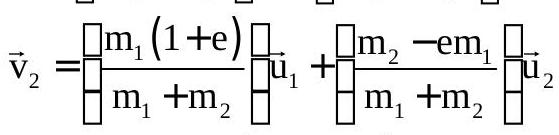268682 A massive ball moving with a speed \(v\) collides head on with a fine ball having mass very much smaller than the mass of the first ball at rest. The collision is elastic and then immediately after the impact, the second ball will move with a speed approximately equal to
268683 A \(1 \mathrm{~kg}\) ball moving at \(12 \mathrm{~m} / \mathrm{s}\) collides head on with a \(2 \mathrm{~kg}\) ball moving in the opposite direction at \(24 \mathrm{~m} / \mathrm{s}\). The velocity of each ball after the impact, if the coefficient of restitution is \(2 / 3\) is
268682 A massive ball moving with a speed \(v\) collides head on with a fine ball having mass very much smaller than the mass of the first ball at rest. The collision is elastic and then immediately after the impact, the second ball will move with a speed approximately equal to
268683 A \(1 \mathrm{~kg}\) ball moving at \(12 \mathrm{~m} / \mathrm{s}\) collides head on with a \(2 \mathrm{~kg}\) ball moving in the opposite direction at \(24 \mathrm{~m} / \mathrm{s}\). The velocity of each ball after the impact, if the coefficient of restitution is \(2 / 3\) is
268682 A massive ball moving with a speed \(v\) collides head on with a fine ball having mass very much smaller than the mass of the first ball at rest. The collision is elastic and then immediately after the impact, the second ball will move with a speed approximately equal to
268683 A \(1 \mathrm{~kg}\) ball moving at \(12 \mathrm{~m} / \mathrm{s}\) collides head on with a \(2 \mathrm{~kg}\) ball moving in the opposite direction at \(24 \mathrm{~m} / \mathrm{s}\). The velocity of each ball after the impact, if the coefficient of restitution is \(2 / 3\) is
268682 A massive ball moving with a speed \(v\) collides head on with a fine ball having mass very much smaller than the mass of the first ball at rest. The collision is elastic and then immediately after the impact, the second ball will move with a speed approximately equal to
268683 A \(1 \mathrm{~kg}\) ball moving at \(12 \mathrm{~m} / \mathrm{s}\) collides head on with a \(2 \mathrm{~kg}\) ball moving in the opposite direction at \(24 \mathrm{~m} / \mathrm{s}\). The velocity of each ball after the impact, if the coefficient of restitution is \(2 / 3\) is