267994 An electric dipole is made up of two particles having charges \(+1 \mu \mathrm{C}\), mass \(1 \mathrm{~kg}\) and other with charge \(-1 \mu \mathrm{C}\) and mass \(1 \mathrm{~kg}\) separated by distance \(1 \mathrm{~m}\). It is in equilibrium in a uniform electric field of \(20 \times 10^{3} \mathrm{~V} / \mathrm{m}\). If the dipole is deflected through angle \(2^{0}\), time taken by it to comeagain in equilibrium is
267995
A point particle of mass\(M\) is attached to one end of a massless rigid non-conducting rod of length L. Another point particle of the same mass is attached to the other end of the rod. The two particle carry charges \(+q\) and - \(q\) respectively. This arrangement is held in a region of a uniform electric field \(E\) such that the rod makes a small angle \(\theta\) (say of about \(5^{\circ}\) ) with the field direction (see figure). The expression for the minimum time needed for the rod to become parallel to the field after it is set free.
267994 An electric dipole is made up of two particles having charges \(+1 \mu \mathrm{C}\), mass \(1 \mathrm{~kg}\) and other with charge \(-1 \mu \mathrm{C}\) and mass \(1 \mathrm{~kg}\) separated by distance \(1 \mathrm{~m}\). It is in equilibrium in a uniform electric field of \(20 \times 10^{3} \mathrm{~V} / \mathrm{m}\). If the dipole is deflected through angle \(2^{0}\), time taken by it to comeagain in equilibrium is
267995
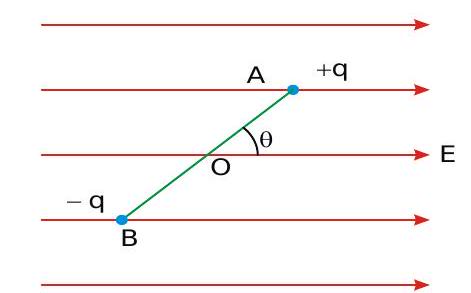
A point particle of mass\(M\) is attached to one end of a massless rigid non-conducting rod of length L. Another point particle of the same mass is attached to the other end of the rod. The two particle carry charges \(+q\) and - \(q\) respectively. This arrangement is held in a region of a uniform electric field \(E\) such that the rod makes a small angle \(\theta\) (say of about \(5^{\circ}\) ) with the field direction (see figure). The expression for the minimum time needed for the rod to become parallel to the field after it is set free.

267994 An electric dipole is made up of two particles having charges \(+1 \mu \mathrm{C}\), mass \(1 \mathrm{~kg}\) and other with charge \(-1 \mu \mathrm{C}\) and mass \(1 \mathrm{~kg}\) separated by distance \(1 \mathrm{~m}\). It is in equilibrium in a uniform electric field of \(20 \times 10^{3} \mathrm{~V} / \mathrm{m}\). If the dipole is deflected through angle \(2^{0}\), time taken by it to comeagain in equilibrium is
267995
A point particle of mass\(M\) is attached to one end of a massless rigid non-conducting rod of length L. Another point particle of the same mass is attached to the other end of the rod. The two particle carry charges \(+q\) and - \(q\) respectively. This arrangement is held in a region of a uniform electric field \(E\) such that the rod makes a small angle \(\theta\) (say of about \(5^{\circ}\) ) with the field direction (see figure). The expression for the minimum time needed for the rod to become parallel to the field after it is set free.

267994 An electric dipole is made up of two particles having charges \(+1 \mu \mathrm{C}\), mass \(1 \mathrm{~kg}\) and other with charge \(-1 \mu \mathrm{C}\) and mass \(1 \mathrm{~kg}\) separated by distance \(1 \mathrm{~m}\). It is in equilibrium in a uniform electric field of \(20 \times 10^{3} \mathrm{~V} / \mathrm{m}\). If the dipole is deflected through angle \(2^{0}\), time taken by it to comeagain in equilibrium is
267995
A point particle of mass\(M\) is attached to one end of a massless rigid non-conducting rod of length L. Another point particle of the same mass is attached to the other end of the rod. The two particle carry charges \(+q\) and - \(q\) respectively. This arrangement is held in a region of a uniform electric field \(E\) such that the rod makes a small angle \(\theta\) (say of about \(5^{\circ}\) ) with the field direction (see figure). The expression for the minimum time needed for the rod to become parallel to the field after it is set free.

267994 An electric dipole is made up of two particles having charges \(+1 \mu \mathrm{C}\), mass \(1 \mathrm{~kg}\) and other with charge \(-1 \mu \mathrm{C}\) and mass \(1 \mathrm{~kg}\) separated by distance \(1 \mathrm{~m}\). It is in equilibrium in a uniform electric field of \(20 \times 10^{3} \mathrm{~V} / \mathrm{m}\). If the dipole is deflected through angle \(2^{0}\), time taken by it to comeagain in equilibrium is
267995
A point particle of mass\(M\) is attached to one end of a massless rigid non-conducting rod of length L. Another point particle of the same mass is attached to the other end of the rod. The two particle carry charges \(+q\) and - \(q\) respectively. This arrangement is held in a region of a uniform electric field \(E\) such that the rod makes a small angle \(\theta\) (say of about \(5^{\circ}\) ) with the field direction (see figure). The expression for the minimum time needed for the rod to become parallel to the field after it is set free.






