142552
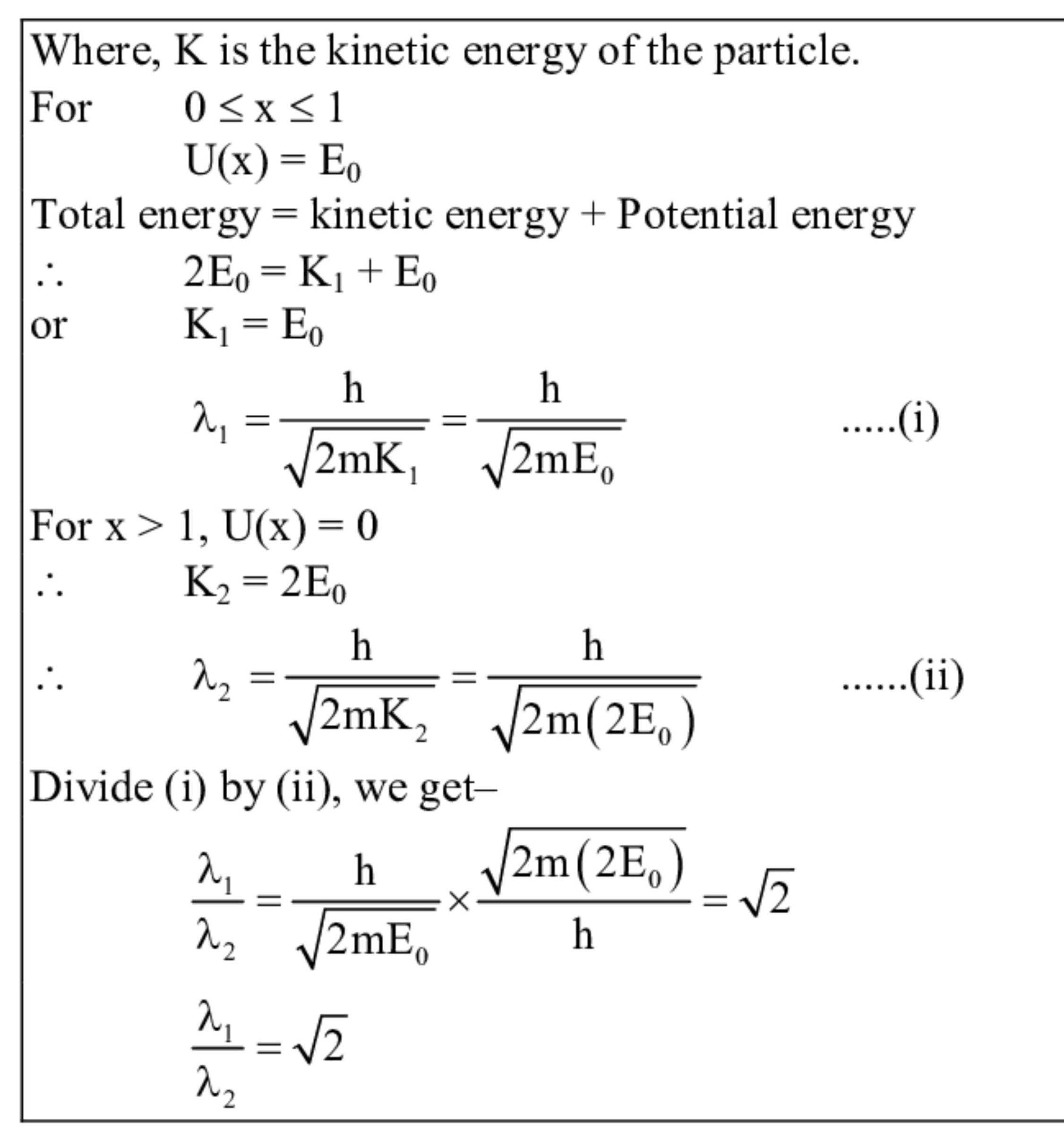
The potential energy of particle of mass $m$ varies as
$U(x)\left\{\begin{array}{c}
E_{0} \text { for } 0 \leq x \leq 1$
$0 \text { for } x>1\right.$
The de Broglie wavelength of the particle in the range $0 \leq x \leq 1$ is $\lambda_{1}$ and that in the range $x>1$ is $\lambda_{2}$. If the total energy of the particle is $2 E_{0}$ find $\lambda_{1} / \lambda_{2}$.
142552
The potential energy of particle of mass $m$ varies as
$U(x)\left\{\begin{array}{c}
E_{0} \text { for } 0 \leq x \leq 1$
$0 \text { for } x>1\right.$
The de Broglie wavelength of the particle in the range $0 \leq x \leq 1$ is $\lambda_{1}$ and that in the range $x>1$ is $\lambda_{2}$. If the total energy of the particle is $2 E_{0}$ find $\lambda_{1} / \lambda_{2}$.
142552
The potential energy of particle of mass $m$ varies as
$U(x)\left\{\begin{array}{c}
E_{0} \text { for } 0 \leq x \leq 1$
$0 \text { for } x>1\right.$
The de Broglie wavelength of the particle in the range $0 \leq x \leq 1$ is $\lambda_{1}$ and that in the range $x>1$ is $\lambda_{2}$. If the total energy of the particle is $2 E_{0}$ find $\lambda_{1} / \lambda_{2}$.
142552
The potential energy of particle of mass $m$ varies as
$U(x)\left\{\begin{array}{c}
E_{0} \text { for } 0 \leq x \leq 1$
$0 \text { for } x>1\right.$
The de Broglie wavelength of the particle in the range $0 \leq x \leq 1$ is $\lambda_{1}$ and that in the range $x>1$ is $\lambda_{2}$. If the total energy of the particle is $2 E_{0}$ find $\lambda_{1} / \lambda_{2}$.
142552
The potential energy of particle of mass $m$ varies as
$U(x)\left\{\begin{array}{c}
E_{0} \text { for } 0 \leq x \leq 1$
$0 \text { for } x>1\right.$
The de Broglie wavelength of the particle in the range $0 \leq x \leq 1$ is $\lambda_{1}$ and that in the range $x>1$ is $\lambda_{2}$. If the total energy of the particle is $2 E_{0}$ find $\lambda_{1} / \lambda_{2}$.