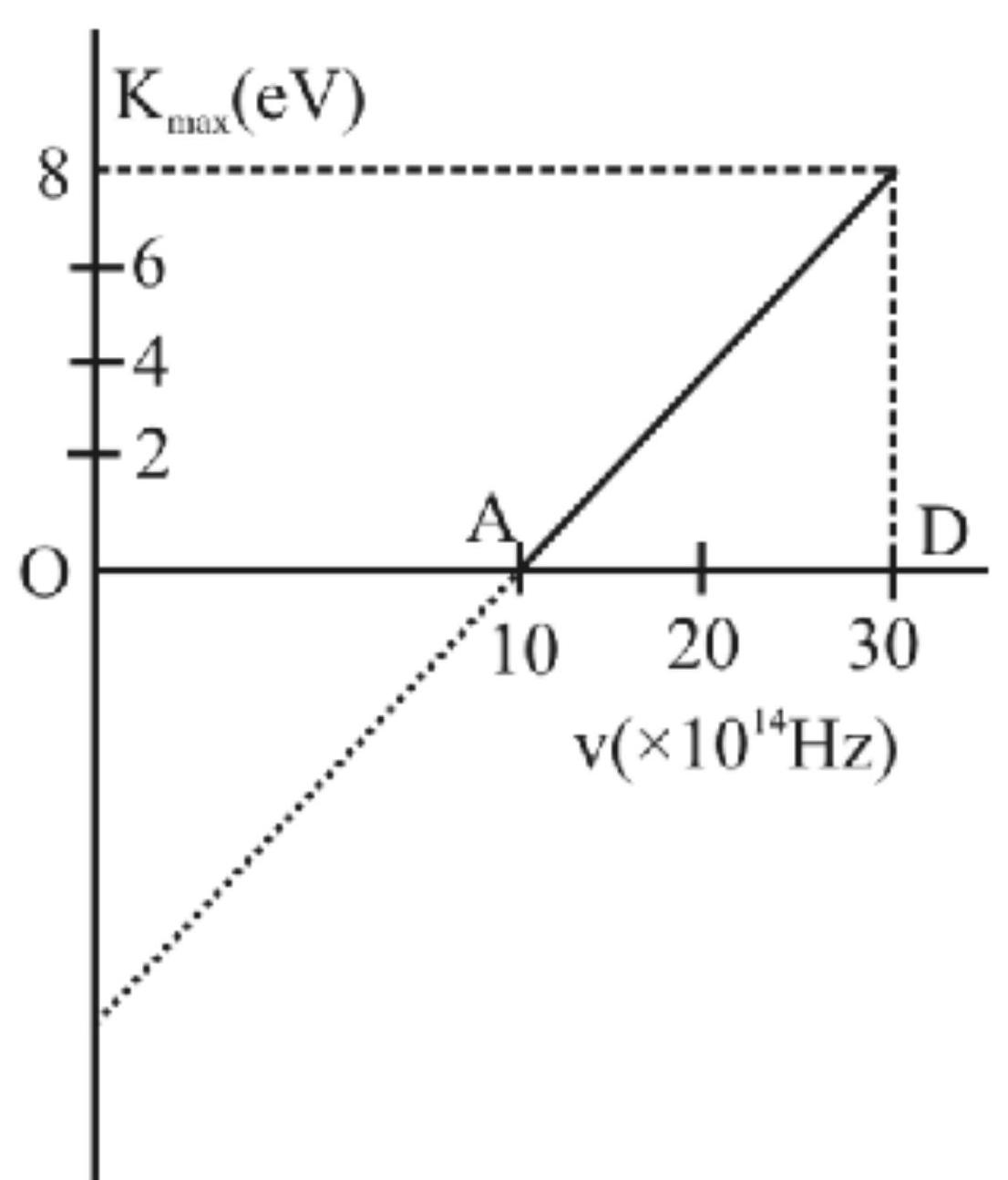
142262 When light of wavelength $300 \mathrm{~nm}$ falls on a photoelectric emitter, photoelectrons are liberated. For another emitter, light of wavelength $600 \mathrm{~nm}$ is sufficient for liberating photoelectrons. The ratio of the work function of the two emitters is
142262 When light of wavelength $300 \mathrm{~nm}$ falls on a photoelectric emitter, photoelectrons are liberated. For another emitter, light of wavelength $600 \mathrm{~nm}$ is sufficient for liberating photoelectrons. The ratio of the work function of the two emitters is
142262 When light of wavelength $300 \mathrm{~nm}$ falls on a photoelectric emitter, photoelectrons are liberated. For another emitter, light of wavelength $600 \mathrm{~nm}$ is sufficient for liberating photoelectrons. The ratio of the work function of the two emitters is
142262 When light of wavelength $300 \mathrm{~nm}$ falls on a photoelectric emitter, photoelectrons are liberated. For another emitter, light of wavelength $600 \mathrm{~nm}$ is sufficient for liberating photoelectrons. The ratio of the work function of the two emitters is