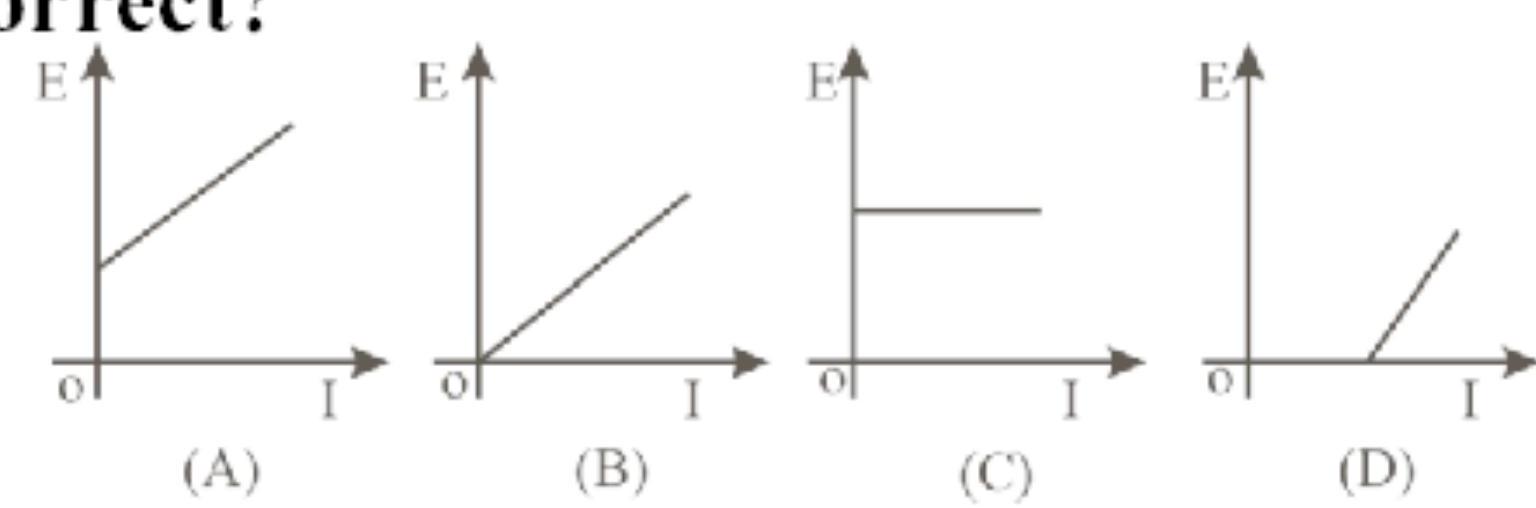
142208 When wavelength of incident radiation on the metal surface is reduced from ' $\lambda_{1}$ ' to ' $\lambda_{2}$ ', the kinetic energy of emitted photoelectrons is tripled. The work function of the metal is $(\mathrm{h}=$ Planck's constant, $\mathrm{c}=$ velocity of light)
142208 When wavelength of incident radiation on the metal surface is reduced from ' $\lambda_{1}$ ' to ' $\lambda_{2}$ ', the kinetic energy of emitted photoelectrons is tripled. The work function of the metal is $(\mathrm{h}=$ Planck's constant, $\mathrm{c}=$ velocity of light)
142208 When wavelength of incident radiation on the metal surface is reduced from ' $\lambda_{1}$ ' to ' $\lambda_{2}$ ', the kinetic energy of emitted photoelectrons is tripled. The work function of the metal is $(\mathrm{h}=$ Planck's constant, $\mathrm{c}=$ velocity of light)
142208 When wavelength of incident radiation on the metal surface is reduced from ' $\lambda_{1}$ ' to ' $\lambda_{2}$ ', the kinetic energy of emitted photoelectrons is tripled. The work function of the metal is $(\mathrm{h}=$ Planck's constant, $\mathrm{c}=$ velocity of light)