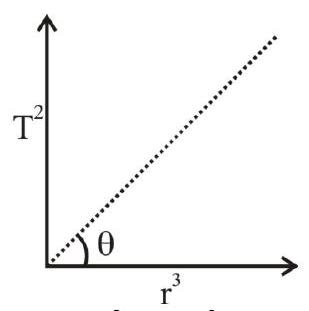
138631 A planet of mass $m$ moves around the Sun along an elliptical path with a period of revolution $T$. During the motion, the planet's maximum and minimum distance from Sun is $R$ and $\frac{R}{3}$ respectively. If $T^{2}=\alpha R^{3}$, then the magnitude of constant $\alpha$ will be
138633 Taking that Earth revolves around the Sun in a circular orbit of radius $15 \times 10^{10} \mathrm{~m}$, with a time period of 1 year. The time taken by another planet, which is at a distance of $540 \times 10^{10} \mathrm{~m}$, to revolve around the Sun in circular orbit once, will be
138631 A planet of mass $m$ moves around the Sun along an elliptical path with a period of revolution $T$. During the motion, the planet's maximum and minimum distance from Sun is $R$ and $\frac{R}{3}$ respectively. If $T^{2}=\alpha R^{3}$, then the magnitude of constant $\alpha$ will be
138633 Taking that Earth revolves around the Sun in a circular orbit of radius $15 \times 10^{10} \mathrm{~m}$, with a time period of 1 year. The time taken by another planet, which is at a distance of $540 \times 10^{10} \mathrm{~m}$, to revolve around the Sun in circular orbit once, will be
138631 A planet of mass $m$ moves around the Sun along an elliptical path with a period of revolution $T$. During the motion, the planet's maximum and minimum distance from Sun is $R$ and $\frac{R}{3}$ respectively. If $T^{2}=\alpha R^{3}$, then the magnitude of constant $\alpha$ will be
138633 Taking that Earth revolves around the Sun in a circular orbit of radius $15 \times 10^{10} \mathrm{~m}$, with a time period of 1 year. The time taken by another planet, which is at a distance of $540 \times 10^{10} \mathrm{~m}$, to revolve around the Sun in circular orbit once, will be
138631 A planet of mass $m$ moves around the Sun along an elliptical path with a period of revolution $T$. During the motion, the planet's maximum and minimum distance from Sun is $R$ and $\frac{R}{3}$ respectively. If $T^{2}=\alpha R^{3}$, then the magnitude of constant $\alpha$ will be
138633 Taking that Earth revolves around the Sun in a circular orbit of radius $15 \times 10^{10} \mathrm{~m}$, with a time period of 1 year. The time taken by another planet, which is at a distance of $540 \times 10^{10} \mathrm{~m}$, to revolve around the Sun in circular orbit once, will be
138631 A planet of mass $m$ moves around the Sun along an elliptical path with a period of revolution $T$. During the motion, the planet's maximum and minimum distance from Sun is $R$ and $\frac{R}{3}$ respectively. If $T^{2}=\alpha R^{3}$, then the magnitude of constant $\alpha$ will be
138633 Taking that Earth revolves around the Sun in a circular orbit of radius $15 \times 10^{10} \mathrm{~m}$, with a time period of 1 year. The time taken by another planet, which is at a distance of $540 \times 10^{10} \mathrm{~m}$, to revolve around the Sun in circular orbit once, will be