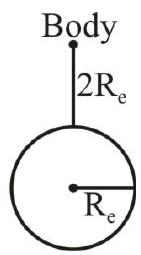
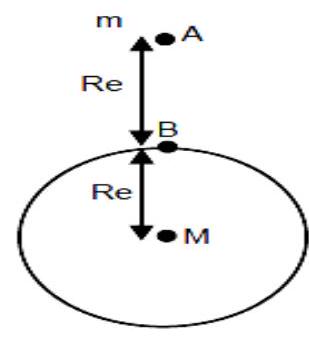
138460 If the gravitational filed in the space is given as $\left(-\frac{K}{r^{2}}\right)$ Taking the reference point to be at $\mathbf{r}=\mathbf{2}$ cm with gravitational potential $V=10 \mathrm{~J} / \mathrm{kg}$. Find the gravitational potential at $r=3 \mathrm{~cm}$ in SI unit (Given that, $K=6 \mathrm{Jcm} / \mathrm{kg}$ )
138463 Infinite number of masses, each $1 \mathrm{~kg}$, are placed along the $x$-axis at $x= \pm 1 \mathrm{~m} . \pm 2 \mathrm{~m} . \pm 4$ $\mathrm{m}, \pm 8 \mathrm{~m}, \pm 16 \mathrm{~m} \ldots .$. The magnitude of the resultant gravitational potential in terms of gravitational constant $G$ at the origin $(x=0)$ is
138460 If the gravitational filed in the space is given as $\left(-\frac{K}{r^{2}}\right)$ Taking the reference point to be at $\mathbf{r}=\mathbf{2}$ cm with gravitational potential $V=10 \mathrm{~J} / \mathrm{kg}$. Find the gravitational potential at $r=3 \mathrm{~cm}$ in SI unit (Given that, $K=6 \mathrm{Jcm} / \mathrm{kg}$ )
138463 Infinite number of masses, each $1 \mathrm{~kg}$, are placed along the $x$-axis at $x= \pm 1 \mathrm{~m} . \pm 2 \mathrm{~m} . \pm 4$ $\mathrm{m}, \pm 8 \mathrm{~m}, \pm 16 \mathrm{~m} \ldots .$. The magnitude of the resultant gravitational potential in terms of gravitational constant $G$ at the origin $(x=0)$ is
138460 If the gravitational filed in the space is given as $\left(-\frac{K}{r^{2}}\right)$ Taking the reference point to be at $\mathbf{r}=\mathbf{2}$ cm with gravitational potential $V=10 \mathrm{~J} / \mathrm{kg}$. Find the gravitational potential at $r=3 \mathrm{~cm}$ in SI unit (Given that, $K=6 \mathrm{Jcm} / \mathrm{kg}$ )
138463 Infinite number of masses, each $1 \mathrm{~kg}$, are placed along the $x$-axis at $x= \pm 1 \mathrm{~m} . \pm 2 \mathrm{~m} . \pm 4$ $\mathrm{m}, \pm 8 \mathrm{~m}, \pm 16 \mathrm{~m} \ldots .$. The magnitude of the resultant gravitational potential in terms of gravitational constant $G$ at the origin $(x=0)$ is
138460 If the gravitational filed in the space is given as $\left(-\frac{K}{r^{2}}\right)$ Taking the reference point to be at $\mathbf{r}=\mathbf{2}$ cm with gravitational potential $V=10 \mathrm{~J} / \mathrm{kg}$. Find the gravitational potential at $r=3 \mathrm{~cm}$ in SI unit (Given that, $K=6 \mathrm{Jcm} / \mathrm{kg}$ )
138463 Infinite number of masses, each $1 \mathrm{~kg}$, are placed along the $x$-axis at $x= \pm 1 \mathrm{~m} . \pm 2 \mathrm{~m} . \pm 4$ $\mathrm{m}, \pm 8 \mathrm{~m}, \pm 16 \mathrm{~m} \ldots .$. The magnitude of the resultant gravitational potential in terms of gravitational constant $G$ at the origin $(x=0)$ is
138460 If the gravitational filed in the space is given as $\left(-\frac{K}{r^{2}}\right)$ Taking the reference point to be at $\mathbf{r}=\mathbf{2}$ cm with gravitational potential $V=10 \mathrm{~J} / \mathrm{kg}$. Find the gravitational potential at $r=3 \mathrm{~cm}$ in SI unit (Given that, $K=6 \mathrm{Jcm} / \mathrm{kg}$ )
138463 Infinite number of masses, each $1 \mathrm{~kg}$, are placed along the $x$-axis at $x= \pm 1 \mathrm{~m} . \pm 2 \mathrm{~m} . \pm 4$ $\mathrm{m}, \pm 8 \mathrm{~m}, \pm 16 \mathrm{~m} \ldots .$. The magnitude of the resultant gravitational potential in terms of gravitational constant $G$ at the origin $(x=0)$ is