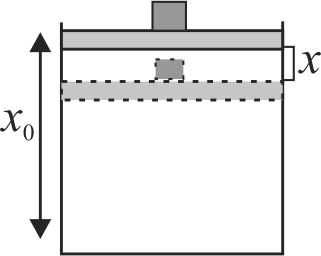
371490 An ideal gas enclosed in a vertical cylindrical container supports a freely moving piston of mass \(M\). The piston and the cylinder have equal cross-sectional area \(A\). When the piston is in equilibrium the volume of the gas is \(V_{0}\) and its pressure is \(P_{0}\). The piston is slightly displaced from the equilibrium position and released. Assuming that the system is completely isolated from its surrounding, the piston executes a simple harmonic motion with frequency.
371490 An ideal gas enclosed in a vertical cylindrical container supports a freely moving piston of mass \(M\). The piston and the cylinder have equal cross-sectional area \(A\). When the piston is in equilibrium the volume of the gas is \(V_{0}\) and its pressure is \(P_{0}\). The piston is slightly displaced from the equilibrium position and released. Assuming that the system is completely isolated from its surrounding, the piston executes a simple harmonic motion with frequency.
371490 An ideal gas enclosed in a vertical cylindrical container supports a freely moving piston of mass \(M\). The piston and the cylinder have equal cross-sectional area \(A\). When the piston is in equilibrium the volume of the gas is \(V_{0}\) and its pressure is \(P_{0}\). The piston is slightly displaced from the equilibrium position and released. Assuming that the system is completely isolated from its surrounding, the piston executes a simple harmonic motion with frequency.
371490 An ideal gas enclosed in a vertical cylindrical container supports a freely moving piston of mass \(M\). The piston and the cylinder have equal cross-sectional area \(A\). When the piston is in equilibrium the volume of the gas is \(V_{0}\) and its pressure is \(P_{0}\). The piston is slightly displaced from the equilibrium position and released. Assuming that the system is completely isolated from its surrounding, the piston executes a simple harmonic motion with frequency.