368042 In Young’s double slit experiment, we get 10 fringes in the field of view of monochromatic light of wavelength \(4000{\rm{ }}\mathop A\limits^ \circ \), then the number of fringes obtained in the same field of view for wavelength \(5000\mathop {{\rm{ }}A}\limits^ \circ \) is
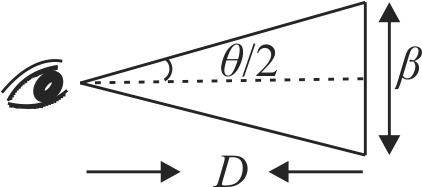
368046 In Young's double slit experiment, the distance between slits and the screen is \(1.0\,m\) and monochromatic light of \(600\,nm\) is being used. A person standing near the slits is looking at the fringe pattern. When the separation between the slits is varied, the interference pattern disappears for a particular distance \(d_{0}\) between the slits. If the angular resolution of the eye is \(\dfrac{1^{\circ}}{60}\) the value of \({d_o}\) is close to
368042 In Young’s double slit experiment, we get 10 fringes in the field of view of monochromatic light of wavelength \(4000{\rm{ }}\mathop A\limits^ \circ \), then the number of fringes obtained in the same field of view for wavelength \(5000\mathop {{\rm{ }}A}\limits^ \circ \) is
368046 In Young's double slit experiment, the distance between slits and the screen is \(1.0\,m\) and monochromatic light of \(600\,nm\) is being used. A person standing near the slits is looking at the fringe pattern. When the separation between the slits is varied, the interference pattern disappears for a particular distance \(d_{0}\) between the slits. If the angular resolution of the eye is \(\dfrac{1^{\circ}}{60}\) the value of \({d_o}\) is close to
368042 In Young’s double slit experiment, we get 10 fringes in the field of view of monochromatic light of wavelength \(4000{\rm{ }}\mathop A\limits^ \circ \), then the number of fringes obtained in the same field of view for wavelength \(5000\mathop {{\rm{ }}A}\limits^ \circ \) is
368046 In Young's double slit experiment, the distance between slits and the screen is \(1.0\,m\) and monochromatic light of \(600\,nm\) is being used. A person standing near the slits is looking at the fringe pattern. When the separation between the slits is varied, the interference pattern disappears for a particular distance \(d_{0}\) between the slits. If the angular resolution of the eye is \(\dfrac{1^{\circ}}{60}\) the value of \({d_o}\) is close to
368042 In Young’s double slit experiment, we get 10 fringes in the field of view of monochromatic light of wavelength \(4000{\rm{ }}\mathop A\limits^ \circ \), then the number of fringes obtained in the same field of view for wavelength \(5000\mathop {{\rm{ }}A}\limits^ \circ \) is
368046 In Young's double slit experiment, the distance between slits and the screen is \(1.0\,m\) and monochromatic light of \(600\,nm\) is being used. A person standing near the slits is looking at the fringe pattern. When the separation between the slits is varied, the interference pattern disappears for a particular distance \(d_{0}\) between the slits. If the angular resolution of the eye is \(\dfrac{1^{\circ}}{60}\) the value of \({d_o}\) is close to
368042 In Young’s double slit experiment, we get 10 fringes in the field of view of monochromatic light of wavelength \(4000{\rm{ }}\mathop A\limits^ \circ \), then the number of fringes obtained in the same field of view for wavelength \(5000\mathop {{\rm{ }}A}\limits^ \circ \) is
368046 In Young's double slit experiment, the distance between slits and the screen is \(1.0\,m\) and monochromatic light of \(600\,nm\) is being used. A person standing near the slits is looking at the fringe pattern. When the separation between the slits is varied, the interference pattern disappears for a particular distance \(d_{0}\) between the slits. If the angular resolution of the eye is \(\dfrac{1^{\circ}}{60}\) the value of \({d_o}\) is close to