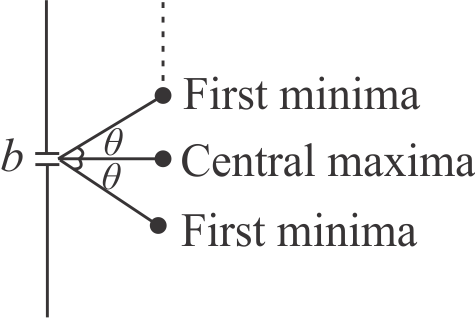
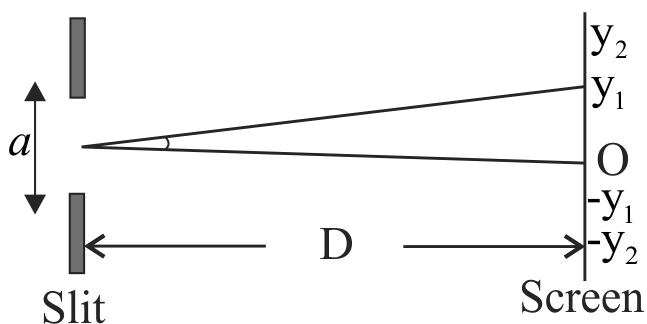
367694 A linear aperture whose width is \(0.02\,cm\) is placed immediately infront of a lens of focal length \(60\,cm\). The aperture is illuminated normally by a parallel beam of wavelength \(5 \times {10^{ - 5}}cm.\) The distance of the first dark band of the diffraction pattern from the centre of the screen is
367694 A linear aperture whose width is \(0.02\,cm\) is placed immediately infront of a lens of focal length \(60\,cm\). The aperture is illuminated normally by a parallel beam of wavelength \(5 \times {10^{ - 5}}cm.\) The distance of the first dark band of the diffraction pattern from the centre of the screen is
367694 A linear aperture whose width is \(0.02\,cm\) is placed immediately infront of a lens of focal length \(60\,cm\). The aperture is illuminated normally by a parallel beam of wavelength \(5 \times {10^{ - 5}}cm.\) The distance of the first dark band of the diffraction pattern from the centre of the screen is
367694 A linear aperture whose width is \(0.02\,cm\) is placed immediately infront of a lens of focal length \(60\,cm\). The aperture is illuminated normally by a parallel beam of wavelength \(5 \times {10^{ - 5}}cm.\) The distance of the first dark band of the diffraction pattern from the centre of the screen is
367694 A linear aperture whose width is \(0.02\,cm\) is placed immediately infront of a lens of focal length \(60\,cm\). The aperture is illuminated normally by a parallel beam of wavelength \(5 \times {10^{ - 5}}cm.\) The distance of the first dark band of the diffraction pattern from the centre of the screen is