363480 A ball of mass 100 \(gm\) is released from a height \({h_1} = 5\,m\) from the ground level and then rebounds to a height \({h_2} = 1.25\,m\). The time of contact of the ball and the ground is \(\Delta t = 0.01\,\sec \).The impulsive force offered by the ball on the ground is :
363483
A body of mass \(1\,kg\) moving with velocity \({1 {~m} / {s}}\) makes an elastic one dimensional collision with an identical stationary body. They are in contact for brief time \(1\,sec\). Their force of interaction increases from zero to \({F_{0}}\) linearly in time \(0.5\,s\) and decreases linearly to zero in further time \(0.5\,sec\) as shown in figure. Find the magnitude of force \({F_{0}}\)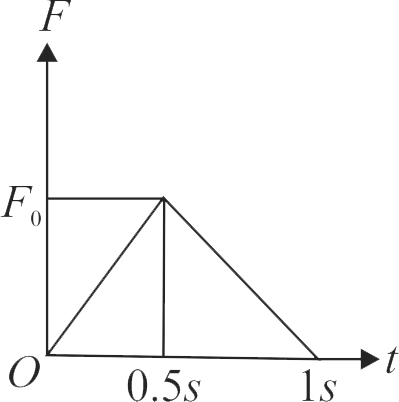
363480 A ball of mass 100 \(gm\) is released from a height \({h_1} = 5\,m\) from the ground level and then rebounds to a height \({h_2} = 1.25\,m\). The time of contact of the ball and the ground is \(\Delta t = 0.01\,\sec \).The impulsive force offered by the ball on the ground is :
363483
A body of mass \(1\,kg\) moving with velocity \({1 {~m} / {s}}\) makes an elastic one dimensional collision with an identical stationary body. They are in contact for brief time \(1\,sec\). Their force of interaction increases from zero to \({F_{0}}\) linearly in time \(0.5\,s\) and decreases linearly to zero in further time \(0.5\,sec\) as shown in figure. Find the magnitude of force \({F_{0}}\)
363480 A ball of mass 100 \(gm\) is released from a height \({h_1} = 5\,m\) from the ground level and then rebounds to a height \({h_2} = 1.25\,m\). The time of contact of the ball and the ground is \(\Delta t = 0.01\,\sec \).The impulsive force offered by the ball on the ground is :
363483
A body of mass \(1\,kg\) moving with velocity \({1 {~m} / {s}}\) makes an elastic one dimensional collision with an identical stationary body. They are in contact for brief time \(1\,sec\). Their force of interaction increases from zero to \({F_{0}}\) linearly in time \(0.5\,s\) and decreases linearly to zero in further time \(0.5\,sec\) as shown in figure. Find the magnitude of force \({F_{0}}\)
363480 A ball of mass 100 \(gm\) is released from a height \({h_1} = 5\,m\) from the ground level and then rebounds to a height \({h_2} = 1.25\,m\). The time of contact of the ball and the ground is \(\Delta t = 0.01\,\sec \).The impulsive force offered by the ball on the ground is :
363483
A body of mass \(1\,kg\) moving with velocity \({1 {~m} / {s}}\) makes an elastic one dimensional collision with an identical stationary body. They are in contact for brief time \(1\,sec\). Their force of interaction increases from zero to \({F_{0}}\) linearly in time \(0.5\,s\) and decreases linearly to zero in further time \(0.5\,sec\) as shown in figure. Find the magnitude of force \({F_{0}}\)
363480 A ball of mass 100 \(gm\) is released from a height \({h_1} = 5\,m\) from the ground level and then rebounds to a height \({h_2} = 1.25\,m\). The time of contact of the ball and the ground is \(\Delta t = 0.01\,\sec \).The impulsive force offered by the ball on the ground is :
363483
A body of mass \(1\,kg\) moving with velocity \({1 {~m} / {s}}\) makes an elastic one dimensional collision with an identical stationary body. They are in contact for brief time \(1\,sec\). Their force of interaction increases from zero to \({F_{0}}\) linearly in time \(0.5\,s\) and decreases linearly to zero in further time \(0.5\,sec\) as shown in figure. Find the magnitude of force \({F_{0}}\)
.png)
