363078
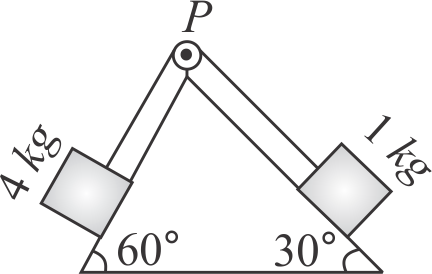
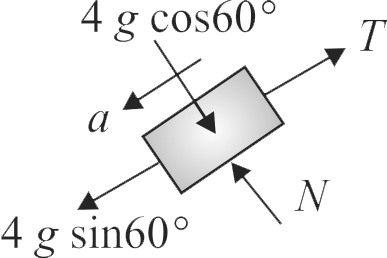
At \(t=0\), the lower end of the bar \(A\) is just above the upper end of bar \(B\) (mass of bar \(A=3 {~kg}\), mass of bar \(B=\dfrac{11}{3} {~kg}\). Find time when upper end of block \(A\) just crosses the lower end of \(B\). (Assume the system was released at \(t=0)\).\(\left(g=10 {~m} / {s}^{2}\right)\)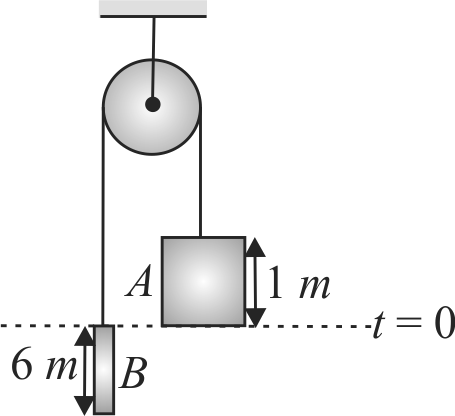
363078
At \(t=0\), the lower end of the bar \(A\) is just above the upper end of bar \(B\) (mass of bar \(A=3 {~kg}\), mass of bar \(B=\dfrac{11}{3} {~kg}\). Find time when upper end of block \(A\) just crosses the lower end of \(B\). (Assume the system was released at \(t=0)\).\(\left(g=10 {~m} / {s}^{2}\right)\)
363078
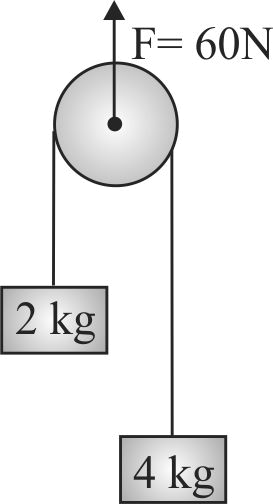
At \(t=0\), the lower end of the bar \(A\) is just above the upper end of bar \(B\) (mass of bar \(A=3 {~kg}\), mass of bar \(B=\dfrac{11}{3} {~kg}\). Find time when upper end of block \(A\) just crosses the lower end of \(B\). (Assume the system was released at \(t=0)\).\(\left(g=10 {~m} / {s}^{2}\right)\)
363078
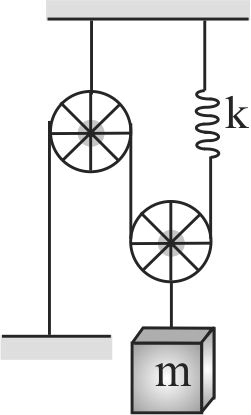
At \(t=0\), the lower end of the bar \(A\) is just above the upper end of bar \(B\) (mass of bar \(A=3 {~kg}\), mass of bar \(B=\dfrac{11}{3} {~kg}\). Find time when upper end of block \(A\) just crosses the lower end of \(B\). (Assume the system was released at \(t=0)\).\(\left(g=10 {~m} / {s}^{2}\right)\)
363078
At \(t=0\), the lower end of the bar \(A\) is just above the upper end of bar \(B\) (mass of bar \(A=3 {~kg}\), mass of bar \(B=\dfrac{11}{3} {~kg}\). Find time when upper end of block \(A\) just crosses the lower end of \(B\). (Assume the system was released at \(t=0)\).\(\left(g=10 {~m} / {s}^{2}\right)\)