363021
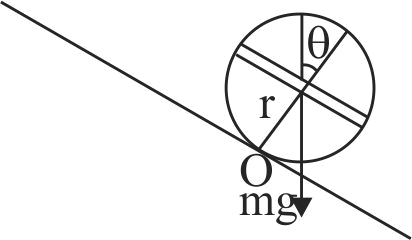
In the figure shown, a coil of single turn is wound on a sphere of radius \(r\) and mass \(m\). The plane of the coil is parallel to the inclined plane and lies in the equatorial plane of the sphere. If the sphere is in rotational equlibrium, the value of \(B\) is (current in the coil is \(i\) )
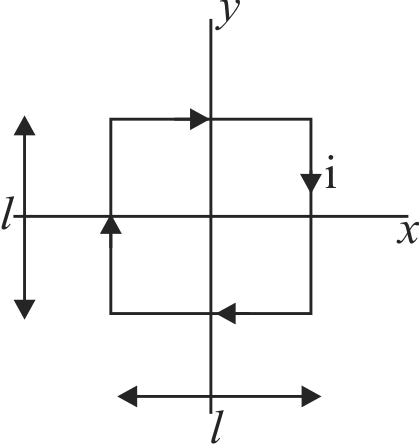
363023 A current \(I\) flows in a rectangulary shaped wire whose center lies at \(\left( {{x_0},0,0} \right)\) and whose vertices are located at the points \(A\left( {{x_0} + d, - a, - b} \right)\), \(B\left( {{x_0} - d,a, - b} \right),\)\(C\left( {{x_0} - d,a,b} \right),\)and \(D\left( {{x_0} + d, - a,b} \right)\) respectively. Assume that \(a,b,d < < {x_0}\). What is the magnitude of magnetic dipole vector of the rectangular wire frame? (Given : \(b = 10\,m,\) \(d = 12\,m,\) \(a = 5\,m,\) \(I = 0.03\,A\,)\)
363021
In the figure shown, a coil of single turn is wound on a sphere of radius \(r\) and mass \(m\). The plane of the coil is parallel to the inclined plane and lies in the equatorial plane of the sphere. If the sphere is in rotational equlibrium, the value of \(B\) is (current in the coil is \(i\) )
363023 A current \(I\) flows in a rectangulary shaped wire whose center lies at \(\left( {{x_0},0,0} \right)\) and whose vertices are located at the points \(A\left( {{x_0} + d, - a, - b} \right)\), \(B\left( {{x_0} - d,a, - b} \right),\)\(C\left( {{x_0} - d,a,b} \right),\)and \(D\left( {{x_0} + d, - a,b} \right)\) respectively. Assume that \(a,b,d < < {x_0}\). What is the magnitude of magnetic dipole vector of the rectangular wire frame? (Given : \(b = 10\,m,\) \(d = 12\,m,\) \(a = 5\,m,\) \(I = 0.03\,A\,)\)
363021
In the figure shown, a coil of single turn is wound on a sphere of radius \(r\) and mass \(m\). The plane of the coil is parallel to the inclined plane and lies in the equatorial plane of the sphere. If the sphere is in rotational equlibrium, the value of \(B\) is (current in the coil is \(i\) )
363023 A current \(I\) flows in a rectangulary shaped wire whose center lies at \(\left( {{x_0},0,0} \right)\) and whose vertices are located at the points \(A\left( {{x_0} + d, - a, - b} \right)\), \(B\left( {{x_0} - d,a, - b} \right),\)\(C\left( {{x_0} - d,a,b} \right),\)and \(D\left( {{x_0} + d, - a,b} \right)\) respectively. Assume that \(a,b,d < < {x_0}\). What is the magnitude of magnetic dipole vector of the rectangular wire frame? (Given : \(b = 10\,m,\) \(d = 12\,m,\) \(a = 5\,m,\) \(I = 0.03\,A\,)\)
363021
In the figure shown, a coil of single turn is wound on a sphere of radius \(r\) and mass \(m\). The plane of the coil is parallel to the inclined plane and lies in the equatorial plane of the sphere. If the sphere is in rotational equlibrium, the value of \(B\) is (current in the coil is \(i\) )
363023 A current \(I\) flows in a rectangulary shaped wire whose center lies at \(\left( {{x_0},0,0} \right)\) and whose vertices are located at the points \(A\left( {{x_0} + d, - a, - b} \right)\), \(B\left( {{x_0} - d,a, - b} \right),\)\(C\left( {{x_0} - d,a,b} \right),\)and \(D\left( {{x_0} + d, - a,b} \right)\) respectively. Assume that \(a,b,d < < {x_0}\). What is the magnitude of magnetic dipole vector of the rectangular wire frame? (Given : \(b = 10\,m,\) \(d = 12\,m,\) \(a = 5\,m,\) \(I = 0.03\,A\,)\)
363021
In the figure shown, a coil of single turn is wound on a sphere of radius \(r\) and mass \(m\). The plane of the coil is parallel to the inclined plane and lies in the equatorial plane of the sphere. If the sphere is in rotational equlibrium, the value of \(B\) is (current in the coil is \(i\) )
363023 A current \(I\) flows in a rectangulary shaped wire whose center lies at \(\left( {{x_0},0,0} \right)\) and whose vertices are located at the points \(A\left( {{x_0} + d, - a, - b} \right)\), \(B\left( {{x_0} - d,a, - b} \right),\)\(C\left( {{x_0} - d,a,b} \right),\)and \(D\left( {{x_0} + d, - a,b} \right)\) respectively. Assume that \(a,b,d < < {x_0}\). What is the magnitude of magnetic dipole vector of the rectangular wire frame? (Given : \(b = 10\,m,\) \(d = 12\,m,\) \(a = 5\,m,\) \(I = 0.03\,A\,)\)