362243 A clock has \(75\;cm,\) \(60\;cm\) long second hand and minute hand, respectively. In 30 minutes duration, the tip of second hand will travel \(x\) distance more than the tip of minute hand. The value of \(x\) in meter is nearly (Take \(\pi=3.14\) )

362244 Mr. Eswar drives his car at uniform speed from bottom of a mountain to the top in 30 minutes. At the beginning the speedometer of his car shows 6225 \(km\), while on reaching the top it reads 6285 \(km\). The total distance covered is

362245 A drunked walking in a narrow lane takes 8 steps forward and 6 steps backward, followed again by 8 steps forward and so on. Each step is 1 \(m\) long and requires 1 \(s\). Determine how long the drunkard takes to fall in a pit 10 \(m\) away from the start.

362246 An athlete completes one round of a circular track of radius \(R\) in 40 sec. What will be his displacement at the end of 2 min 20 sec?

362247 A length of path \({A C B}\) is \(1500\,m\) and the length of the path \({A D B}\) is \(2100\,m\). Two particles start from point \({A}\) simultaneously around the track \({A C B D A}\). One of them travels the track in clockwise sense and other in anticlockwise sense with their respective constant speeds. After \(12\,s\) from the start, the first time they meet at the point \({B}\). After minimum time (in \(s\)) in which they meet first at point \({B}\), will they again meet at the point \({B}\) is time \({t_{\min }=(12)^{x} {~s}}\). The value of \({x}\) is

362243 A clock has \(75\;cm,\) \(60\;cm\) long second hand and minute hand, respectively. In 30 minutes duration, the tip of second hand will travel \(x\) distance more than the tip of minute hand. The value of \(x\) in meter is nearly (Take \(\pi=3.14\) )

362244 Mr. Eswar drives his car at uniform speed from bottom of a mountain to the top in 30 minutes. At the beginning the speedometer of his car shows 6225 \(km\), while on reaching the top it reads 6285 \(km\). The total distance covered is

362245 A drunked walking in a narrow lane takes 8 steps forward and 6 steps backward, followed again by 8 steps forward and so on. Each step is 1 \(m\) long and requires 1 \(s\). Determine how long the drunkard takes to fall in a pit 10 \(m\) away from the start.

362246 An athlete completes one round of a circular track of radius \(R\) in 40 sec. What will be his displacement at the end of 2 min 20 sec?

362247 A length of path \({A C B}\) is \(1500\,m\) and the length of the path \({A D B}\) is \(2100\,m\). Two particles start from point \({A}\) simultaneously around the track \({A C B D A}\). One of them travels the track in clockwise sense and other in anticlockwise sense with their respective constant speeds. After \(12\,s\) from the start, the first time they meet at the point \({B}\). After minimum time (in \(s\)) in which they meet first at point \({B}\), will they again meet at the point \({B}\) is time \({t_{\min }=(12)^{x} {~s}}\). The value of \({x}\) is

362243 A clock has \(75\;cm,\) \(60\;cm\) long second hand and minute hand, respectively. In 30 minutes duration, the tip of second hand will travel \(x\) distance more than the tip of minute hand. The value of \(x\) in meter is nearly (Take \(\pi=3.14\) )

362244 Mr. Eswar drives his car at uniform speed from bottom of a mountain to the top in 30 minutes. At the beginning the speedometer of his car shows 6225 \(km\), while on reaching the top it reads 6285 \(km\). The total distance covered is

362245 A drunked walking in a narrow lane takes 8 steps forward and 6 steps backward, followed again by 8 steps forward and so on. Each step is 1 \(m\) long and requires 1 \(s\). Determine how long the drunkard takes to fall in a pit 10 \(m\) away from the start.

362246 An athlete completes one round of a circular track of radius \(R\) in 40 sec. What will be his displacement at the end of 2 min 20 sec?

362247 A length of path \({A C B}\) is \(1500\,m\) and the length of the path \({A D B}\) is \(2100\,m\). Two particles start from point \({A}\) simultaneously around the track \({A C B D A}\). One of them travels the track in clockwise sense and other in anticlockwise sense with their respective constant speeds. After \(12\,s\) from the start, the first time they meet at the point \({B}\). After minimum time (in \(s\)) in which they meet first at point \({B}\), will they again meet at the point \({B}\) is time \({t_{\min }=(12)^{x} {~s}}\). The value of \({x}\) is

362243 A clock has \(75\;cm,\) \(60\;cm\) long second hand and minute hand, respectively. In 30 minutes duration, the tip of second hand will travel \(x\) distance more than the tip of minute hand. The value of \(x\) in meter is nearly (Take \(\pi=3.14\) )

362244 Mr. Eswar drives his car at uniform speed from bottom of a mountain to the top in 30 minutes. At the beginning the speedometer of his car shows 6225 \(km\), while on reaching the top it reads 6285 \(km\). The total distance covered is

362245 A drunked walking in a narrow lane takes 8 steps forward and 6 steps backward, followed again by 8 steps forward and so on. Each step is 1 \(m\) long and requires 1 \(s\). Determine how long the drunkard takes to fall in a pit 10 \(m\) away from the start.

362246 An athlete completes one round of a circular track of radius \(R\) in 40 sec. What will be his displacement at the end of 2 min 20 sec?

362247 A length of path \({A C B}\) is \(1500\,m\) and the length of the path \({A D B}\) is \(2100\,m\). Two particles start from point \({A}\) simultaneously around the track \({A C B D A}\). One of them travels the track in clockwise sense and other in anticlockwise sense with their respective constant speeds. After \(12\,s\) from the start, the first time they meet at the point \({B}\). After minimum time (in \(s\)) in which they meet first at point \({B}\), will they again meet at the point \({B}\) is time \({t_{\min }=(12)^{x} {~s}}\). The value of \({x}\) is

362243 A clock has \(75\;cm,\) \(60\;cm\) long second hand and minute hand, respectively. In 30 minutes duration, the tip of second hand will travel \(x\) distance more than the tip of minute hand. The value of \(x\) in meter is nearly (Take \(\pi=3.14\) )

362244 Mr. Eswar drives his car at uniform speed from bottom of a mountain to the top in 30 minutes. At the beginning the speedometer of his car shows 6225 \(km\), while on reaching the top it reads 6285 \(km\). The total distance covered is

362245 A drunked walking in a narrow lane takes 8 steps forward and 6 steps backward, followed again by 8 steps forward and so on. Each step is 1 \(m\) long and requires 1 \(s\). Determine how long the drunkard takes to fall in a pit 10 \(m\) away from the start.

362246 An athlete completes one round of a circular track of radius \(R\) in 40 sec. What will be his displacement at the end of 2 min 20 sec?

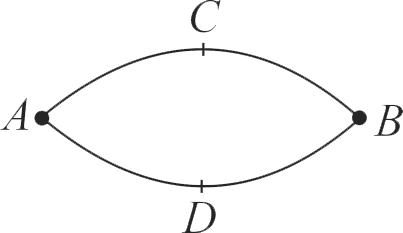
362247 A length of path \({A C B}\) is \(1500\,m\) and the length of the path \({A D B}\) is \(2100\,m\). Two particles start from point \({A}\) simultaneously around the track \({A C B D A}\). One of them travels the track in clockwise sense and other in anticlockwise sense with their respective constant speeds. After \(12\,s\) from the start, the first time they meet at the point \({B}\). After minimum time (in \(s\)) in which they meet first at point \({B}\), will they again meet at the point \({B}\) is time \({t_{\min }=(12)^{x} {~s}}\). The value of \({x}\) is