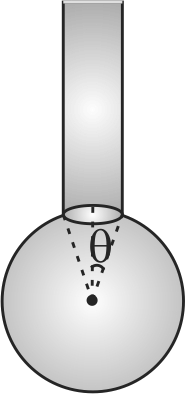
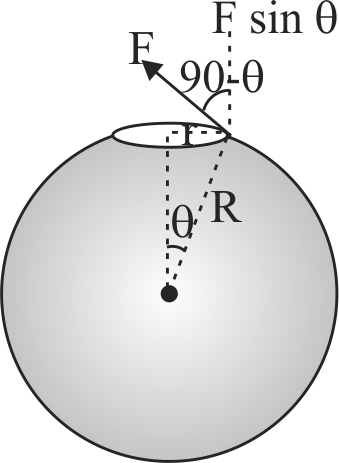
361221 When liquid medicine of density \(\rho\) is to be put in the eye, it is done with the help of a dropper. As the bulb on the top of the dropper is pressed, a drop forms at the opening of the dropper. We wish to estimate the size of the drop. We first assume that the drop formed at the opening is spherical because that requires a minimum increase in its surface energy. To determine the size, we calculate the net vertical force due to the surface tension \(T\) when the radius of the drop is \(R\). When the force becomes smaller than the weight of the drop, the drop gets detached from the dropper. If the radius of the opening of the dropper is \(r\), the vertical force due to the surface tension on the drop of radius \(R\) (assuming \(r < < R\)) is
361221 When liquid medicine of density \(\rho\) is to be put in the eye, it is done with the help of a dropper. As the bulb on the top of the dropper is pressed, a drop forms at the opening of the dropper. We wish to estimate the size of the drop. We first assume that the drop formed at the opening is spherical because that requires a minimum increase in its surface energy. To determine the size, we calculate the net vertical force due to the surface tension \(T\) when the radius of the drop is \(R\). When the force becomes smaller than the weight of the drop, the drop gets detached from the dropper. If the radius of the opening of the dropper is \(r\), the vertical force due to the surface tension on the drop of radius \(R\) (assuming \(r < < R\)) is
361221 When liquid medicine of density \(\rho\) is to be put in the eye, it is done with the help of a dropper. As the bulb on the top of the dropper is pressed, a drop forms at the opening of the dropper. We wish to estimate the size of the drop. We first assume that the drop formed at the opening is spherical because that requires a minimum increase in its surface energy. To determine the size, we calculate the net vertical force due to the surface tension \(T\) when the radius of the drop is \(R\). When the force becomes smaller than the weight of the drop, the drop gets detached from the dropper. If the radius of the opening of the dropper is \(r\), the vertical force due to the surface tension on the drop of radius \(R\) (assuming \(r < < R\)) is
361221 When liquid medicine of density \(\rho\) is to be put in the eye, it is done with the help of a dropper. As the bulb on the top of the dropper is pressed, a drop forms at the opening of the dropper. We wish to estimate the size of the drop. We first assume that the drop formed at the opening is spherical because that requires a minimum increase in its surface energy. To determine the size, we calculate the net vertical force due to the surface tension \(T\) when the radius of the drop is \(R\). When the force becomes smaller than the weight of the drop, the drop gets detached from the dropper. If the radius of the opening of the dropper is \(r\), the vertical force due to the surface tension on the drop of radius \(R\) (assuming \(r < < R\)) is