355720 A pilot of mass \(m\) can bear a maximum apparent weight 7 times of \(m g\). The aeroplane is moving in a vertical circle. If the velocity of aeroplane is \(210\;m{\rm{/}}s\) while diving up from the lowest point of vertical circle, the minimum radius of vertical circle should be
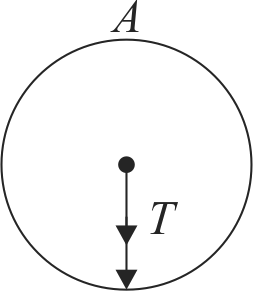
355721 One end of a string of length \(1.0\;m\) is tied to a body of mass \(0.5\;kg.\) It is whirled in a vertical circle with angular frequency \(4\,rad{s^{ - 1}}.\) The tension in the string when the body is at the lower most point of its motion will be equal to (take, \(g = 10\;m{s^{ - 2}}\) )
355720 A pilot of mass \(m\) can bear a maximum apparent weight 7 times of \(m g\). The aeroplane is moving in a vertical circle. If the velocity of aeroplane is \(210\;m{\rm{/}}s\) while diving up from the lowest point of vertical circle, the minimum radius of vertical circle should be
355721 One end of a string of length \(1.0\;m\) is tied to a body of mass \(0.5\;kg.\) It is whirled in a vertical circle with angular frequency \(4\,rad{s^{ - 1}}.\) The tension in the string when the body is at the lower most point of its motion will be equal to (take, \(g = 10\;m{s^{ - 2}}\) )
355720 A pilot of mass \(m\) can bear a maximum apparent weight 7 times of \(m g\). The aeroplane is moving in a vertical circle. If the velocity of aeroplane is \(210\;m{\rm{/}}s\) while diving up from the lowest point of vertical circle, the minimum radius of vertical circle should be
355721 One end of a string of length \(1.0\;m\) is tied to a body of mass \(0.5\;kg.\) It is whirled in a vertical circle with angular frequency \(4\,rad{s^{ - 1}}.\) The tension in the string when the body is at the lower most point of its motion will be equal to (take, \(g = 10\;m{s^{ - 2}}\) )
355720 A pilot of mass \(m\) can bear a maximum apparent weight 7 times of \(m g\). The aeroplane is moving in a vertical circle. If the velocity of aeroplane is \(210\;m{\rm{/}}s\) while diving up from the lowest point of vertical circle, the minimum radius of vertical circle should be
355721 One end of a string of length \(1.0\;m\) is tied to a body of mass \(0.5\;kg.\) It is whirled in a vertical circle with angular frequency \(4\,rad{s^{ - 1}}.\) The tension in the string when the body is at the lower most point of its motion will be equal to (take, \(g = 10\;m{s^{ - 2}}\) )