355264
A boy throws a ball with speed \(u\) in a well of depth 14 \(m\) as shown. On bounce with bottom of the well the speed of the ball gets halved. What should be the minimum value of \(u\) such that the ball may be able to reach his hand again? Assume that his hands are at 1 \(m\) height from top of the well while throwing and catching.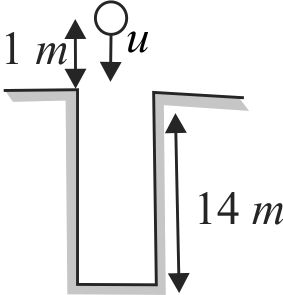
355267
The friction coefficient between the horizontal surface and blocks \({A}\) and \({B}\) are \({\dfrac{1}{15}}\) and \({\dfrac{2}{15}}\) respectively. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest.
355264
A boy throws a ball with speed \(u\) in a well of depth 14 \(m\) as shown. On bounce with bottom of the well the speed of the ball gets halved. What should be the minimum value of \(u\) such that the ball may be able to reach his hand again? Assume that his hands are at 1 \(m\) height from top of the well while throwing and catching.
355267
The friction coefficient between the horizontal surface and blocks \({A}\) and \({B}\) are \({\dfrac{1}{15}}\) and \({\dfrac{2}{15}}\) respectively. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest.
355264
A boy throws a ball with speed \(u\) in a well of depth 14 \(m\) as shown. On bounce with bottom of the well the speed of the ball gets halved. What should be the minimum value of \(u\) such that the ball may be able to reach his hand again? Assume that his hands are at 1 \(m\) height from top of the well while throwing and catching.
355267
The friction coefficient between the horizontal surface and blocks \({A}\) and \({B}\) are \({\dfrac{1}{15}}\) and \({\dfrac{2}{15}}\) respectively. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest.
355264
A boy throws a ball with speed \(u\) in a well of depth 14 \(m\) as shown. On bounce with bottom of the well the speed of the ball gets halved. What should be the minimum value of \(u\) such that the ball may be able to reach his hand again? Assume that his hands are at 1 \(m\) height from top of the well while throwing and catching.
355267
The friction coefficient between the horizontal surface and blocks \({A}\) and \({B}\) are \({\dfrac{1}{15}}\) and \({\dfrac{2}{15}}\) respectively. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest.
355264
A boy throws a ball with speed \(u\) in a well of depth 14 \(m\) as shown. On bounce with bottom of the well the speed of the ball gets halved. What should be the minimum value of \(u\) such that the ball may be able to reach his hand again? Assume that his hands are at 1 \(m\) height from top of the well while throwing and catching.
355267
The friction coefficient between the horizontal surface and blocks \({A}\) and \({B}\) are \({\dfrac{1}{15}}\) and \({\dfrac{2}{15}}\) respectively. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest.

