355050
\(S_{1}\) and \(S_{2}\) are two coherent sources of sound having no initial phase difference. The velocity of sound is \(330\;m/s\). No minima will be formed on the line passing through \(S_{2}\) and perpendicular to the line joining \(S_{1}\) and \(S_{2}\), if the frequency of both the sources may be :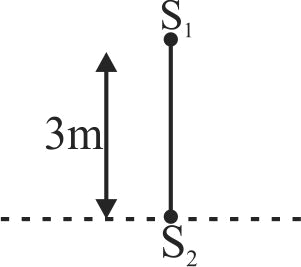
355053
Sound signal is sent through a composite tube as shown in Fig. The radius of the semicircle is \(r\). Speed of sound in air is \(v\). The source of sound is capable to generate frequencies in the range \(f_{1}\) to \(f_{2}\left(f_{2}>f_{1}\right)\). If \(n\) is an integer then frequency for maximum intensity at ' \(p\) ' is given by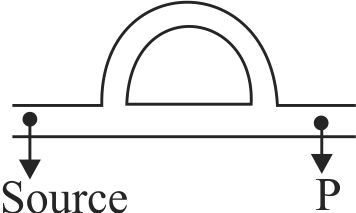
355050
\(S_{1}\) and \(S_{2}\) are two coherent sources of sound having no initial phase difference. The velocity of sound is \(330\;m/s\). No minima will be formed on the line passing through \(S_{2}\) and perpendicular to the line joining \(S_{1}\) and \(S_{2}\), if the frequency of both the sources may be :
355053
Sound signal is sent through a composite tube as shown in Fig. The radius of the semicircle is \(r\). Speed of sound in air is \(v\). The source of sound is capable to generate frequencies in the range \(f_{1}\) to \(f_{2}\left(f_{2}>f_{1}\right)\). If \(n\) is an integer then frequency for maximum intensity at ' \(p\) ' is given by
355050
\(S_{1}\) and \(S_{2}\) are two coherent sources of sound having no initial phase difference. The velocity of sound is \(330\;m/s\). No minima will be formed on the line passing through \(S_{2}\) and perpendicular to the line joining \(S_{1}\) and \(S_{2}\), if the frequency of both the sources may be :
355053
Sound signal is sent through a composite tube as shown in Fig. The radius of the semicircle is \(r\). Speed of sound in air is \(v\). The source of sound is capable to generate frequencies in the range \(f_{1}\) to \(f_{2}\left(f_{2}>f_{1}\right)\). If \(n\) is an integer then frequency for maximum intensity at ' \(p\) ' is given by
355050
\(S_{1}\) and \(S_{2}\) are two coherent sources of sound having no initial phase difference. The velocity of sound is \(330\;m/s\). No minima will be formed on the line passing through \(S_{2}\) and perpendicular to the line joining \(S_{1}\) and \(S_{2}\), if the frequency of both the sources may be :
355053
Sound signal is sent through a composite tube as shown in Fig. The radius of the semicircle is \(r\). Speed of sound in air is \(v\). The source of sound is capable to generate frequencies in the range \(f_{1}\) to \(f_{2}\left(f_{2}>f_{1}\right)\). If \(n\) is an integer then frequency for maximum intensity at ' \(p\) ' is given by
355050
\(S_{1}\) and \(S_{2}\) are two coherent sources of sound having no initial phase difference. The velocity of sound is \(330\;m/s\). No minima will be formed on the line passing through \(S_{2}\) and perpendicular to the line joining \(S_{1}\) and \(S_{2}\), if the frequency of both the sources may be :
355053
Sound signal is sent through a composite tube as shown in Fig. The radius of the semicircle is \(r\). Speed of sound in air is \(v\). The source of sound is capable to generate frequencies in the range \(f_{1}\) to \(f_{2}\left(f_{2}>f_{1}\right)\). If \(n\) is an integer then frequency for maximum intensity at ' \(p\) ' is given by