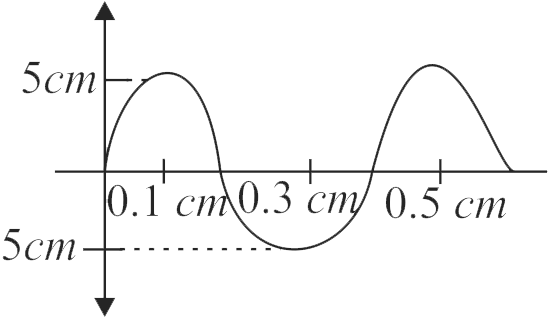354569
A progressive wave on a string having linear mass density \({\rho}\) is represented by
\({y=A \sin \left(\dfrac{2 \pi}{\lambda} x-\omega t\right)}\) where \({y}\) is in \({m m}\). Find the total energy (in \({\mu J}\) ) passing through origin from \({t=0}\) to \({t=\dfrac{\pi}{2 \omega}}\).
[Take: \({\rho=3 \times 10^{-2} {~kg} / {m} ; A=1 {~mm}}\); \({\omega=100 {rad} / {sec} ; \lambda=16 {~cm}]}\)
354569
A progressive wave on a string having linear mass density \({\rho}\) is represented by
\({y=A \sin \left(\dfrac{2 \pi}{\lambda} x-\omega t\right)}\) where \({y}\) is in \({m m}\). Find the total energy (in \({\mu J}\) ) passing through origin from \({t=0}\) to \({t=\dfrac{\pi}{2 \omega}}\).
[Take: \({\rho=3 \times 10^{-2} {~kg} / {m} ; A=1 {~mm}}\); \({\omega=100 {rad} / {sec} ; \lambda=16 {~cm}]}\)
354569
A progressive wave on a string having linear mass density \({\rho}\) is represented by
\({y=A \sin \left(\dfrac{2 \pi}{\lambda} x-\omega t\right)}\) where \({y}\) is in \({m m}\). Find the total energy (in \({\mu J}\) ) passing through origin from \({t=0}\) to \({t=\dfrac{\pi}{2 \omega}}\).
[Take: \({\rho=3 \times 10^{-2} {~kg} / {m} ; A=1 {~mm}}\); \({\omega=100 {rad} / {sec} ; \lambda=16 {~cm}]}\)
354569
A progressive wave on a string having linear mass density \({\rho}\) is represented by
\({y=A \sin \left(\dfrac{2 \pi}{\lambda} x-\omega t\right)}\) where \({y}\) is in \({m m}\). Find the total energy (in \({\mu J}\) ) passing through origin from \({t=0}\) to \({t=\dfrac{\pi}{2 \omega}}\).
[Take: \({\rho=3 \times 10^{-2} {~kg} / {m} ; A=1 {~mm}}\); \({\omega=100 {rad} / {sec} ; \lambda=16 {~cm}]}\)
354569
A progressive wave on a string having linear mass density \({\rho}\) is represented by
\({y=A \sin \left(\dfrac{2 \pi}{\lambda} x-\omega t\right)}\) where \({y}\) is in \({m m}\). Find the total energy (in \({\mu J}\) ) passing through origin from \({t=0}\) to \({t=\dfrac{\pi}{2 \omega}}\).
[Take: \({\rho=3 \times 10^{-2} {~kg} / {m} ; A=1 {~mm}}\); \({\omega=100 {rad} / {sec} ; \lambda=16 {~cm}]}\)