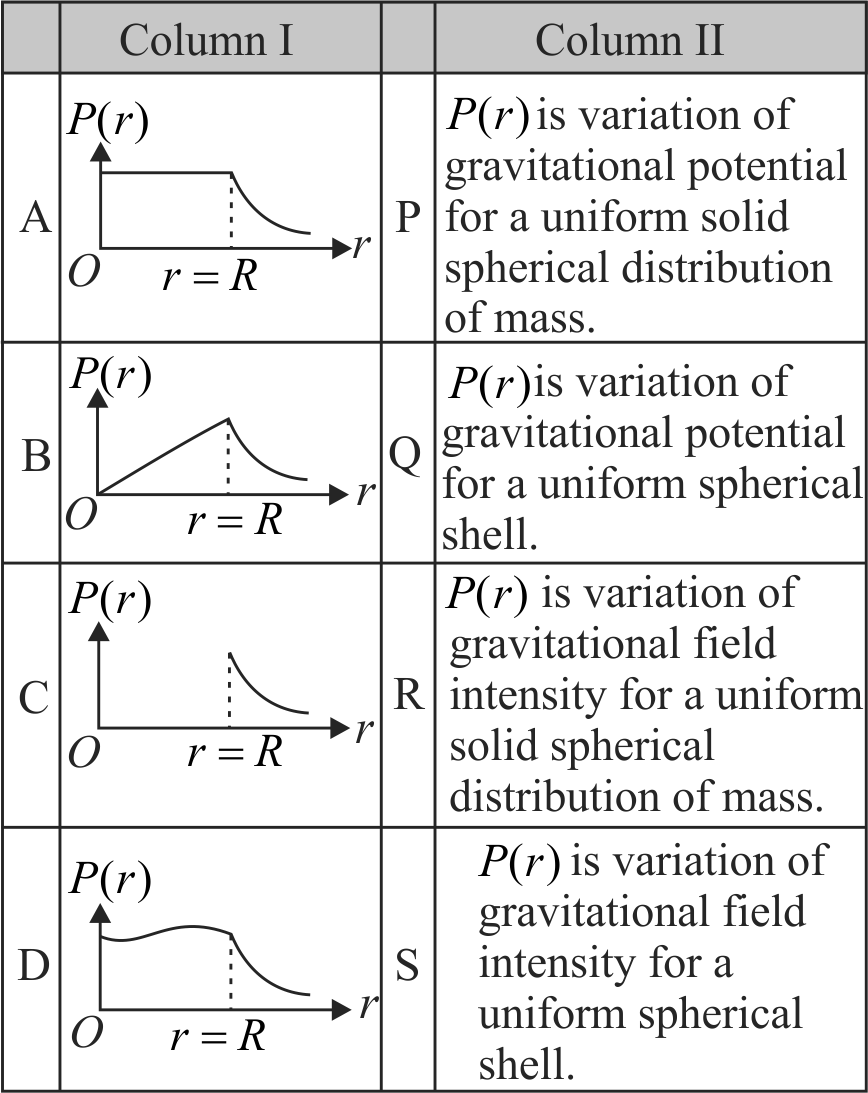
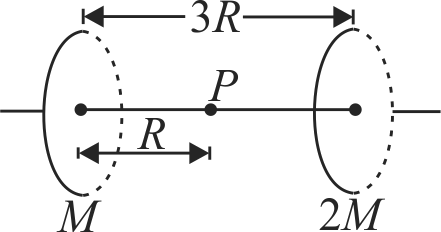
359876 The gravitational potential at a point above the surface of earth is \( - 5.12 \times {10^7}\;J/kg\) and the acceleration due to gravity at that point is \(6.4\;m/{s^2}.\) Assume that the mean radius of earth to be \(6400\,km\) . The height of this point above the earth's surface is
359876 The gravitational potential at a point above the surface of earth is \( - 5.12 \times {10^7}\;J/kg\) and the acceleration due to gravity at that point is \(6.4\;m/{s^2}.\) Assume that the mean radius of earth to be \(6400\,km\) . The height of this point above the earth's surface is
359876 The gravitational potential at a point above the surface of earth is \( - 5.12 \times {10^7}\;J/kg\) and the acceleration due to gravity at that point is \(6.4\;m/{s^2}.\) Assume that the mean radius of earth to be \(6400\,km\) . The height of this point above the earth's surface is
359876 The gravitational potential at a point above the surface of earth is \( - 5.12 \times {10^7}\;J/kg\) and the acceleration due to gravity at that point is \(6.4\;m/{s^2}.\) Assume that the mean radius of earth to be \(6400\,km\) . The height of this point above the earth's surface is
359876 The gravitational potential at a point above the surface of earth is \( - 5.12 \times {10^7}\;J/kg\) and the acceleration due to gravity at that point is \(6.4\;m/{s^2}.\) Assume that the mean radius of earth to be \(6400\,km\) . The height of this point above the earth's surface is