366254
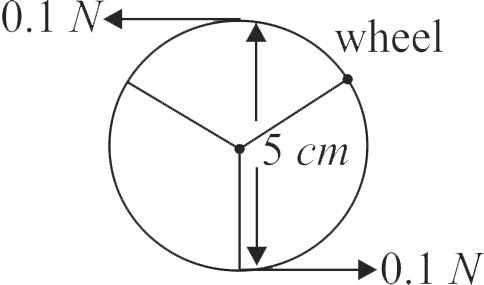
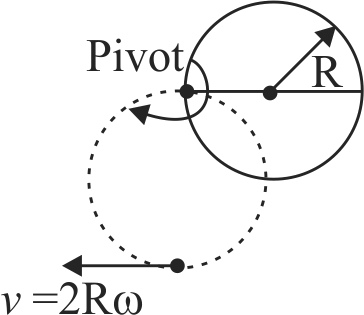
A uniform solid disc of radius \(R\) and mass \(\mathrm{M}\) is free to rotate on a frictionless pivot through a point on its rim. If the disc is released from rest in the position shown in the figure the speed of lowest point on the disc in the dashed position is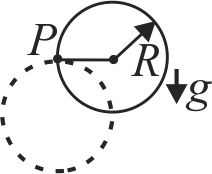
366254
A uniform solid disc of radius \(R\) and mass \(\mathrm{M}\) is free to rotate on a frictionless pivot through a point on its rim. If the disc is released from rest in the position shown in the figure the speed of lowest point on the disc in the dashed position is
366254
A uniform solid disc of radius \(R\) and mass \(\mathrm{M}\) is free to rotate on a frictionless pivot through a point on its rim. If the disc is released from rest in the position shown in the figure the speed of lowest point on the disc in the dashed position is
366254
A uniform solid disc of radius \(R\) and mass \(\mathrm{M}\) is free to rotate on a frictionless pivot through a point on its rim. If the disc is released from rest in the position shown in the figure the speed of lowest point on the disc in the dashed position is