366150
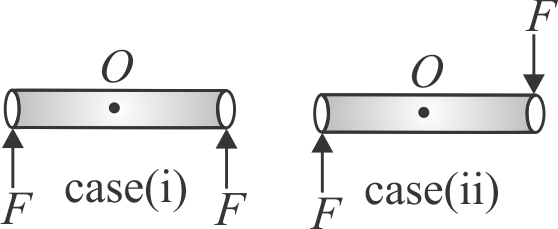
A rigid massless rod \(AB\) of length \(1\;m\) is placed horizontally on two rigid supports at its ends as shown in figure. A weight \(10\;N\) is hung from a point \(C\) at a distance \(30\;cm\) from \(A\). Find the reactions at the supports \(A\) and \(B\) respectively.
366150
A rigid massless rod \(AB\) of length \(1\;m\) is placed horizontally on two rigid supports at its ends as shown in figure. A weight \(10\;N\) is hung from a point \(C\) at a distance \(30\;cm\) from \(A\). Find the reactions at the supports \(A\) and \(B\) respectively.
366150
A rigid massless rod \(AB\) of length \(1\;m\) is placed horizontally on two rigid supports at its ends as shown in figure. A weight \(10\;N\) is hung from a point \(C\) at a distance \(30\;cm\) from \(A\). Find the reactions at the supports \(A\) and \(B\) respectively.
366150
A rigid massless rod \(AB\) of length \(1\;m\) is placed horizontally on two rigid supports at its ends as shown in figure. A weight \(10\;N\) is hung from a point \(C\) at a distance \(30\;cm\) from \(A\). Find the reactions at the supports \(A\) and \(B\) respectively.