365989
A uniform disc of mass \(M\) and radius \(R\), is resting on a table on its rim. The coeeficient of friction between disc and table is \(\mu\). Now the disc is pulled with a force \(F\) as shown in the figure. What is the maximum value of \(F\) for which the disc rolls without slipping?
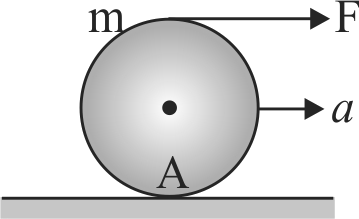
365990 A uniform solid sphere of mass \(m\) is being pulled on a horizontal surface with force \(F\) parallel to the surface and applied at its topmost point. If the acceleration of the sphere is \(a\) & it is in pure rolling on the surface, the value of \(F\) is
365992
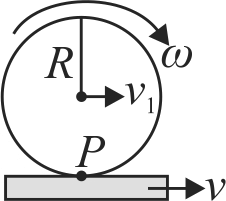
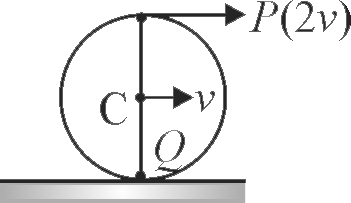
A wheel of a bullock cart is rolling on a level road as shown in the figure below. If its linear speed is \({v}\) in the direction shown, which one of the following options is correct ( \({P}\) and \({Q}\) are any highest and lowest points on the wheel, respectively)?
365989
A uniform disc of mass \(M\) and radius \(R\), is resting on a table on its rim. The coeeficient of friction between disc and table is \(\mu\). Now the disc is pulled with a force \(F\) as shown in the figure. What is the maximum value of \(F\) for which the disc rolls without slipping?
365990 A uniform solid sphere of mass \(m\) is being pulled on a horizontal surface with force \(F\) parallel to the surface and applied at its topmost point. If the acceleration of the sphere is \(a\) & it is in pure rolling on the surface, the value of \(F\) is
365992
A wheel of a bullock cart is rolling on a level road as shown in the figure below. If its linear speed is \({v}\) in the direction shown, which one of the following options is correct ( \({P}\) and \({Q}\) are any highest and lowest points on the wheel, respectively)?
365989
A uniform disc of mass \(M\) and radius \(R\), is resting on a table on its rim. The coeeficient of friction between disc and table is \(\mu\). Now the disc is pulled with a force \(F\) as shown in the figure. What is the maximum value of \(F\) for which the disc rolls without slipping?
365990 A uniform solid sphere of mass \(m\) is being pulled on a horizontal surface with force \(F\) parallel to the surface and applied at its topmost point. If the acceleration of the sphere is \(a\) & it is in pure rolling on the surface, the value of \(F\) is
365992
A wheel of a bullock cart is rolling on a level road as shown in the figure below. If its linear speed is \({v}\) in the direction shown, which one of the following options is correct ( \({P}\) and \({Q}\) are any highest and lowest points on the wheel, respectively)?
365989
A uniform disc of mass \(M\) and radius \(R\), is resting on a table on its rim. The coeeficient of friction between disc and table is \(\mu\). Now the disc is pulled with a force \(F\) as shown in the figure. What is the maximum value of \(F\) for which the disc rolls without slipping?
365990 A uniform solid sphere of mass \(m\) is being pulled on a horizontal surface with force \(F\) parallel to the surface and applied at its topmost point. If the acceleration of the sphere is \(a\) & it is in pure rolling on the surface, the value of \(F\) is
365992
A wheel of a bullock cart is rolling on a level road as shown in the figure below. If its linear speed is \({v}\) in the direction shown, which one of the following options is correct ( \({P}\) and \({Q}\) are any highest and lowest points on the wheel, respectively)?
365989
A uniform disc of mass \(M\) and radius \(R\), is resting on a table on its rim. The coeeficient of friction between disc and table is \(\mu\). Now the disc is pulled with a force \(F\) as shown in the figure. What is the maximum value of \(F\) for which the disc rolls without slipping?
365990 A uniform solid sphere of mass \(m\) is being pulled on a horizontal surface with force \(F\) parallel to the surface and applied at its topmost point. If the acceleration of the sphere is \(a\) & it is in pure rolling on the surface, the value of \(F\) is
365992
A wheel of a bullock cart is rolling on a level road as shown in the figure below. If its linear speed is \({v}\) in the direction shown, which one of the following options is correct ( \({P}\) and \({Q}\) are any highest and lowest points on the wheel, respectively)?