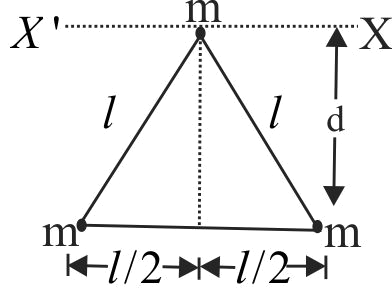
365897 Three points masses, each of mass \(m\) are placed at the corners of an equilateral triangle of side \(l\). The moment of inertia of the system about an axis passing through one of the vertices and parallel to the side joining other two vertices, will be
365897 Three points masses, each of mass \(m\) are placed at the corners of an equilateral triangle of side \(l\). The moment of inertia of the system about an axis passing through one of the vertices and parallel to the side joining other two vertices, will be
365897 Three points masses, each of mass \(m\) are placed at the corners of an equilateral triangle of side \(l\). The moment of inertia of the system about an axis passing through one of the vertices and parallel to the side joining other two vertices, will be
365897 Three points masses, each of mass \(m\) are placed at the corners of an equilateral triangle of side \(l\). The moment of inertia of the system about an axis passing through one of the vertices and parallel to the side joining other two vertices, will be