365659 A disc of moment of interia \('{I_1}'\) is rotating in horizontal plane about an axis passing through its centre and perpendicular to its plane with constant angular speed \('{\omega _1}'\). Another disc of moment of interia \('{I_2}'\) having zero angular speed is placed co - axially on a rotating disc. Now, both the discs are rotating with constant angular speed \('{\omega _2}'\). The energy lost by the initial rotating disc is
365661
Assertion :
When moment of inertia of a rotating body changes, its angular momentum remain conserved, (in the absence of external torque) but its kinetic energy changes.
Reason :
Angular momentum does not depend upon moment of inertia of the body.
365662
A disc of mass ' \(m\) ' and radius ' \(R\) ' is free to rotate in a horizontal plane about a vertical smooth fixed axis passing through its centre. There is a smooth groove along the diameter of the disc and two small balls of mass \(m/2\) each are placed in it on either side of the centre of the disc as shown in the figure. The disc is given an initial angular velocity \(\omega_{0}\) and released.
The angular speed of the disc when the balls reach the end of the disc is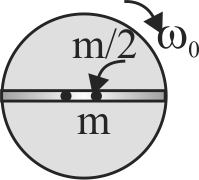
365659 A disc of moment of interia \('{I_1}'\) is rotating in horizontal plane about an axis passing through its centre and perpendicular to its plane with constant angular speed \('{\omega _1}'\). Another disc of moment of interia \('{I_2}'\) having zero angular speed is placed co - axially on a rotating disc. Now, both the discs are rotating with constant angular speed \('{\omega _2}'\). The energy lost by the initial rotating disc is
365661
Assertion :
When moment of inertia of a rotating body changes, its angular momentum remain conserved, (in the absence of external torque) but its kinetic energy changes.
Reason :
Angular momentum does not depend upon moment of inertia of the body.
365662
A disc of mass ' \(m\) ' and radius ' \(R\) ' is free to rotate in a horizontal plane about a vertical smooth fixed axis passing through its centre. There is a smooth groove along the diameter of the disc and two small balls of mass \(m/2\) each are placed in it on either side of the centre of the disc as shown in the figure. The disc is given an initial angular velocity \(\omega_{0}\) and released.
The angular speed of the disc when the balls reach the end of the disc is
365659 A disc of moment of interia \('{I_1}'\) is rotating in horizontal plane about an axis passing through its centre and perpendicular to its plane with constant angular speed \('{\omega _1}'\). Another disc of moment of interia \('{I_2}'\) having zero angular speed is placed co - axially on a rotating disc. Now, both the discs are rotating with constant angular speed \('{\omega _2}'\). The energy lost by the initial rotating disc is
365661
Assertion :
When moment of inertia of a rotating body changes, its angular momentum remain conserved, (in the absence of external torque) but its kinetic energy changes.
Reason :
Angular momentum does not depend upon moment of inertia of the body.
365662
A disc of mass ' \(m\) ' and radius ' \(R\) ' is free to rotate in a horizontal plane about a vertical smooth fixed axis passing through its centre. There is a smooth groove along the diameter of the disc and two small balls of mass \(m/2\) each are placed in it on either side of the centre of the disc as shown in the figure. The disc is given an initial angular velocity \(\omega_{0}\) and released.
The angular speed of the disc when the balls reach the end of the disc is
365659 A disc of moment of interia \('{I_1}'\) is rotating in horizontal plane about an axis passing through its centre and perpendicular to its plane with constant angular speed \('{\omega _1}'\). Another disc of moment of interia \('{I_2}'\) having zero angular speed is placed co - axially on a rotating disc. Now, both the discs are rotating with constant angular speed \('{\omega _2}'\). The energy lost by the initial rotating disc is
365661
Assertion :
When moment of inertia of a rotating body changes, its angular momentum remain conserved, (in the absence of external torque) but its kinetic energy changes.
Reason :
Angular momentum does not depend upon moment of inertia of the body.
365662
A disc of mass ' \(m\) ' and radius ' \(R\) ' is free to rotate in a horizontal plane about a vertical smooth fixed axis passing through its centre. There is a smooth groove along the diameter of the disc and two small balls of mass \(m/2\) each are placed in it on either side of the centre of the disc as shown in the figure. The disc is given an initial angular velocity \(\omega_{0}\) and released.
The angular speed of the disc when the balls reach the end of the disc is
365659 A disc of moment of interia \('{I_1}'\) is rotating in horizontal plane about an axis passing through its centre and perpendicular to its plane with constant angular speed \('{\omega _1}'\). Another disc of moment of interia \('{I_2}'\) having zero angular speed is placed co - axially on a rotating disc. Now, both the discs are rotating with constant angular speed \('{\omega _2}'\). The energy lost by the initial rotating disc is
365661
Assertion :
When moment of inertia of a rotating body changes, its angular momentum remain conserved, (in the absence of external torque) but its kinetic energy changes.
Reason :
Angular momentum does not depend upon moment of inertia of the body.
365662
A disc of mass ' \(m\) ' and radius ' \(R\) ' is free to rotate in a horizontal plane about a vertical smooth fixed axis passing through its centre. There is a smooth groove along the diameter of the disc and two small balls of mass \(m/2\) each are placed in it on either side of the centre of the disc as shown in the figure. The disc is given an initial angular velocity \(\omega_{0}\) and released.
The angular speed of the disc when the balls reach the end of the disc is