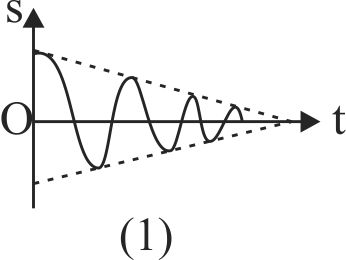
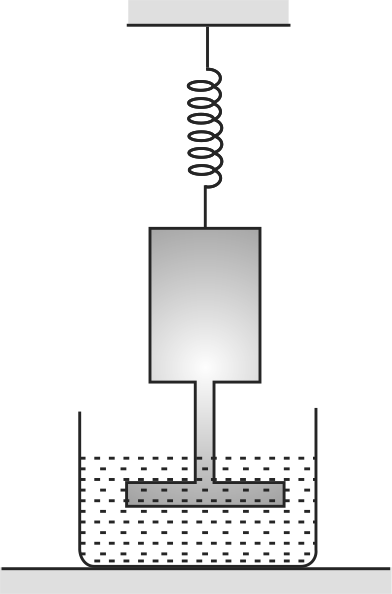
364064 Assume that you are examining the characteristics of the suspension system of a \(2000\;kg\) automobile. The suspension "sags" \(25\,cm\) when the weight of the entire automobile is placed on it. In addition, the amplitude of oscillation decreases by \(50 \%\) in \(100\,\sec \). Estimate the value of \(b\) for the shock absorber system of one wheel, assuming each wheel supports \(500\;kg\).
364064 Assume that you are examining the characteristics of the suspension system of a \(2000\;kg\) automobile. The suspension "sags" \(25\,cm\) when the weight of the entire automobile is placed on it. In addition, the amplitude of oscillation decreases by \(50 \%\) in \(100\,\sec \). Estimate the value of \(b\) for the shock absorber system of one wheel, assuming each wheel supports \(500\;kg\).
364064 Assume that you are examining the characteristics of the suspension system of a \(2000\;kg\) automobile. The suspension "sags" \(25\,cm\) when the weight of the entire automobile is placed on it. In addition, the amplitude of oscillation decreases by \(50 \%\) in \(100\,\sec \). Estimate the value of \(b\) for the shock absorber system of one wheel, assuming each wheel supports \(500\;kg\).
364064 Assume that you are examining the characteristics of the suspension system of a \(2000\;kg\) automobile. The suspension "sags" \(25\,cm\) when the weight of the entire automobile is placed on it. In addition, the amplitude of oscillation decreases by \(50 \%\) in \(100\,\sec \). Estimate the value of \(b\) for the shock absorber system of one wheel, assuming each wheel supports \(500\;kg\).
364064 Assume that you are examining the characteristics of the suspension system of a \(2000\;kg\) automobile. The suspension "sags" \(25\,cm\) when the weight of the entire automobile is placed on it. In addition, the amplitude of oscillation decreases by \(50 \%\) in \(100\,\sec \). Estimate the value of \(b\) for the shock absorber system of one wheel, assuming each wheel supports \(500\;kg\).