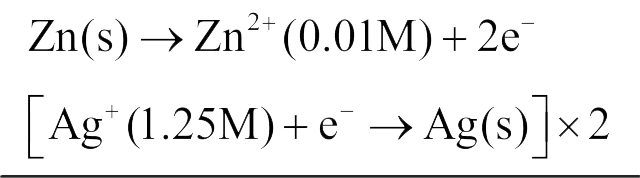330446
The \({{\rm{E}}^{\rm{o}}}\,\,{\rm{at}}\,\,{\rm{25^\circ C}}\) for the following reaction is 0.22 V. Calculate the equilibrium constant at \({\rm{25^\circ C}}\):
\({H_2}\left( g \right) + 2AgCl\left( s \right) \to 2Ag\left( s \right) + 2HCl\left( {aq} \right)\)
Given \({\left( {{\rm{10}}} \right)^{{\rm{7}}{\rm{.44}}}}{\rm{ = 2}}{\rm{.8 \times 1}}{{\rm{0}}^{\rm{7}}}{\rm{,log3 = 0}}{\rm{.4771)}}\)
330449
The logarithm of the equilibrium constant of the cell reaction corresponding to the cell
\({\rm{X(s)}}\left \vert {{{\rm{X}}^{2 + }}({\rm{aq}})} \right \vert \left \vert {{{\rm{Y}}^ + }({\rm{aq}})} \right \vert {\rm{Y(s)}}\) with standard cell potential, \({\rm{E}}{^\circ _{{\rm{cell}}}} = 1.2\;{\rm{V}}\) is given by
330446
The \({{\rm{E}}^{\rm{o}}}\,\,{\rm{at}}\,\,{\rm{25^\circ C}}\) for the following reaction is 0.22 V. Calculate the equilibrium constant at \({\rm{25^\circ C}}\):
\({H_2}\left( g \right) + 2AgCl\left( s \right) \to 2Ag\left( s \right) + 2HCl\left( {aq} \right)\)
Given \({\left( {{\rm{10}}} \right)^{{\rm{7}}{\rm{.44}}}}{\rm{ = 2}}{\rm{.8 \times 1}}{{\rm{0}}^{\rm{7}}}{\rm{,log3 = 0}}{\rm{.4771)}}\)
330449
The logarithm of the equilibrium constant of the cell reaction corresponding to the cell
\({\rm{X(s)}}\left \vert {{{\rm{X}}^{2 + }}({\rm{aq}})} \right \vert \left \vert {{{\rm{Y}}^ + }({\rm{aq}})} \right \vert {\rm{Y(s)}}\) with standard cell potential, \({\rm{E}}{^\circ _{{\rm{cell}}}} = 1.2\;{\rm{V}}\) is given by
330446
The \({{\rm{E}}^{\rm{o}}}\,\,{\rm{at}}\,\,{\rm{25^\circ C}}\) for the following reaction is 0.22 V. Calculate the equilibrium constant at \({\rm{25^\circ C}}\):
\({H_2}\left( g \right) + 2AgCl\left( s \right) \to 2Ag\left( s \right) + 2HCl\left( {aq} \right)\)
Given \({\left( {{\rm{10}}} \right)^{{\rm{7}}{\rm{.44}}}}{\rm{ = 2}}{\rm{.8 \times 1}}{{\rm{0}}^{\rm{7}}}{\rm{,log3 = 0}}{\rm{.4771)}}\)
330449
The logarithm of the equilibrium constant of the cell reaction corresponding to the cell
\({\rm{X(s)}}\left \vert {{{\rm{X}}^{2 + }}({\rm{aq}})} \right \vert \left \vert {{{\rm{Y}}^ + }({\rm{aq}})} \right \vert {\rm{Y(s)}}\) with standard cell potential, \({\rm{E}}{^\circ _{{\rm{cell}}}} = 1.2\;{\rm{V}}\) is given by
330446
The \({{\rm{E}}^{\rm{o}}}\,\,{\rm{at}}\,\,{\rm{25^\circ C}}\) for the following reaction is 0.22 V. Calculate the equilibrium constant at \({\rm{25^\circ C}}\):
\({H_2}\left( g \right) + 2AgCl\left( s \right) \to 2Ag\left( s \right) + 2HCl\left( {aq} \right)\)
Given \({\left( {{\rm{10}}} \right)^{{\rm{7}}{\rm{.44}}}}{\rm{ = 2}}{\rm{.8 \times 1}}{{\rm{0}}^{\rm{7}}}{\rm{,log3 = 0}}{\rm{.4771)}}\)
330449
The logarithm of the equilibrium constant of the cell reaction corresponding to the cell
\({\rm{X(s)}}\left \vert {{{\rm{X}}^{2 + }}({\rm{aq}})} \right \vert \left \vert {{{\rm{Y}}^ + }({\rm{aq}})} \right \vert {\rm{Y(s)}}\) with standard cell potential, \({\rm{E}}{^\circ _{{\rm{cell}}}} = 1.2\;{\rm{V}}\) is given by