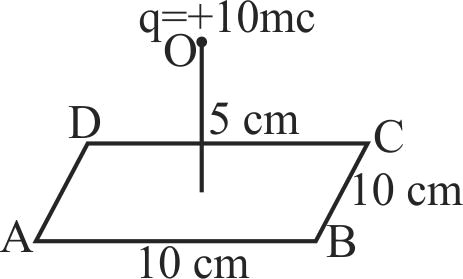
358273 A square surface of side \(L\) metre in the plane of the paper is placed in a uniform electric field \(E\) acting along the same place at an angle \(\theta \) with the horizontal side of the square as shown in figure. The electric flux linked to the surface in unit of \(V\) -\(m\) is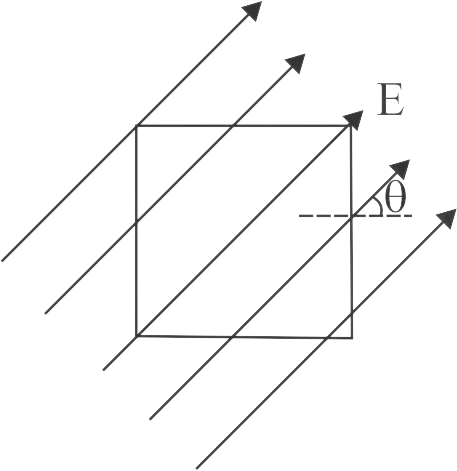

358274 A uniform electric field \(E = 2 \times {10^3}N{C^{ - 1}}\) is acting along the positive \(X\)-axis. The flux of this field through a square of 10 \(cm\) side whose plane is parallel to the \(YZ\) plane is

358275 If \({E=\hat{i}+2 \hat{j}+3 \hat{k}}\), then electric flux (in \({V-m}\) ) through a surface area of \({100 m^{2}}\), lying in the \({X Y}\) plane is

358276 A uniform electric field \({E=3 \times 10^{5} N C^{-1}}\) is acting along the positive Y -axis. The electric flux through a rectangle of area \({10 {~cm} \times 30 {~cm}}\) whose plane is parallel to the \({{Z}-{X}}\) plane is

358277 A point charge \( + 10\mu C\) is at a distance 5\(cm\) directly above the centre of a square of side 10 \(cm\) as shown in the figure
What is the magnitude of the electric flux through the square ? (in \(N{m^2}{C^{ - 1}}\))

358273 A square surface of side \(L\) metre in the plane of the paper is placed in a uniform electric field \(E\) acting along the same place at an angle \(\theta \) with the horizontal side of the square as shown in figure. The electric flux linked to the surface in unit of \(V\) -\(m\) is

358274 A uniform electric field \(E = 2 \times {10^3}N{C^{ - 1}}\) is acting along the positive \(X\)-axis. The flux of this field through a square of 10 \(cm\) side whose plane is parallel to the \(YZ\) plane is

358275 If \({E=\hat{i}+2 \hat{j}+3 \hat{k}}\), then electric flux (in \({V-m}\) ) through a surface area of \({100 m^{2}}\), lying in the \({X Y}\) plane is

358276 A uniform electric field \({E=3 \times 10^{5} N C^{-1}}\) is acting along the positive Y -axis. The electric flux through a rectangle of area \({10 {~cm} \times 30 {~cm}}\) whose plane is parallel to the \({{Z}-{X}}\) plane is

358277 A point charge \( + 10\mu C\) is at a distance 5\(cm\) directly above the centre of a square of side 10 \(cm\) as shown in the figure
What is the magnitude of the electric flux through the square ? (in \(N{m^2}{C^{ - 1}}\))

358273 A square surface of side \(L\) metre in the plane of the paper is placed in a uniform electric field \(E\) acting along the same place at an angle \(\theta \) with the horizontal side of the square as shown in figure. The electric flux linked to the surface in unit of \(V\) -\(m\) is

358274 A uniform electric field \(E = 2 \times {10^3}N{C^{ - 1}}\) is acting along the positive \(X\)-axis. The flux of this field through a square of 10 \(cm\) side whose plane is parallel to the \(YZ\) plane is

358275 If \({E=\hat{i}+2 \hat{j}+3 \hat{k}}\), then electric flux (in \({V-m}\) ) through a surface area of \({100 m^{2}}\), lying in the \({X Y}\) plane is

358276 A uniform electric field \({E=3 \times 10^{5} N C^{-1}}\) is acting along the positive Y -axis. The electric flux through a rectangle of area \({10 {~cm} \times 30 {~cm}}\) whose plane is parallel to the \({{Z}-{X}}\) plane is

358277 A point charge \( + 10\mu C\) is at a distance 5\(cm\) directly above the centre of a square of side 10 \(cm\) as shown in the figure
What is the magnitude of the electric flux through the square ? (in \(N{m^2}{C^{ - 1}}\))

358273 A square surface of side \(L\) metre in the plane of the paper is placed in a uniform electric field \(E\) acting along the same place at an angle \(\theta \) with the horizontal side of the square as shown in figure. The electric flux linked to the surface in unit of \(V\) -\(m\) is

358274 A uniform electric field \(E = 2 \times {10^3}N{C^{ - 1}}\) is acting along the positive \(X\)-axis. The flux of this field through a square of 10 \(cm\) side whose plane is parallel to the \(YZ\) plane is

358275 If \({E=\hat{i}+2 \hat{j}+3 \hat{k}}\), then electric flux (in \({V-m}\) ) through a surface area of \({100 m^{2}}\), lying in the \({X Y}\) plane is

358276 A uniform electric field \({E=3 \times 10^{5} N C^{-1}}\) is acting along the positive Y -axis. The electric flux through a rectangle of area \({10 {~cm} \times 30 {~cm}}\) whose plane is parallel to the \({{Z}-{X}}\) plane is

358277 A point charge \( + 10\mu C\) is at a distance 5\(cm\) directly above the centre of a square of side 10 \(cm\) as shown in the figure
What is the magnitude of the electric flux through the square ? (in \(N{m^2}{C^{ - 1}}\))

358273 A square surface of side \(L\) metre in the plane of the paper is placed in a uniform electric field \(E\) acting along the same place at an angle \(\theta \) with the horizontal side of the square as shown in figure. The electric flux linked to the surface in unit of \(V\) -\(m\) is

358274 A uniform electric field \(E = 2 \times {10^3}N{C^{ - 1}}\) is acting along the positive \(X\)-axis. The flux of this field through a square of 10 \(cm\) side whose plane is parallel to the \(YZ\) plane is

358275 If \({E=\hat{i}+2 \hat{j}+3 \hat{k}}\), then electric flux (in \({V-m}\) ) through a surface area of \({100 m^{2}}\), lying in the \({X Y}\) plane is

358276 A uniform electric field \({E=3 \times 10^{5} N C^{-1}}\) is acting along the positive Y -axis. The electric flux through a rectangle of area \({10 {~cm} \times 30 {~cm}}\) whose plane is parallel to the \({{Z}-{X}}\) plane is

358277 A point charge \( + 10\mu C\) is at a distance 5\(cm\) directly above the centre of a square of side 10 \(cm\) as shown in the figure
What is the magnitude of the electric flux through the square ? (in \(N{m^2}{C^{ - 1}}\))