Explanation:
\(P=100\, \Omega, Q=10\, \Omega, R=300\, \Omega, S=30\, \Omega\)
In the figure, \(I_{1}=\dfrac{330 I}{330+110}=\dfrac{3}{4} I\)
\(I_{2}=I-I_{1}=I-\dfrac{3}{4} I=\dfrac{I}{4} \)
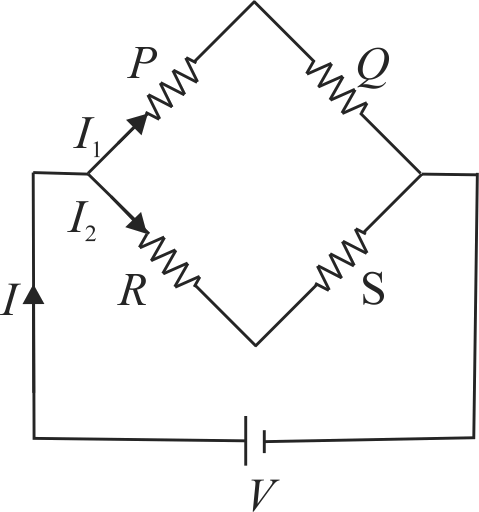
Ratio of heat developed per second across \(P, Q\), \(R\) and \(S,\)
\({H_P}:{H_Q}:{H_R}:{H_S}\)
\( = {\left( {\frac{3}{4}I} \right)^2} \times 100:{\left( {\frac{3}{4}I} \right)^2} \times 10:{\left( {\frac{I}{4}} \right)^2} \times 300\)
\(\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,:{\left( {\frac{I}{4}} \right)^2} \times 30\)
\( = 90:9:30:3 = 30:3:10:1\)