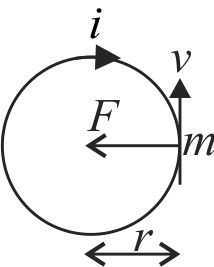
356387
Assuming the atom is in the ground state, the expression for the magnetic field at a point nucleus in hydrogen atom due to circular motion of elecrton is
[ \(\mu_{0} \rightarrow\) permeability of free space,
\(m \rightarrow\) mass of electrons]
\(\left[\epsilon_{0} \rightarrow\right.\) permittivity of free space,
\(h \rightarrow\) planck's constant]
356387
Assuming the atom is in the ground state, the expression for the magnetic field at a point nucleus in hydrogen atom due to circular motion of elecrton is
[ \(\mu_{0} \rightarrow\) permeability of free space,
\(m \rightarrow\) mass of electrons]
\(\left[\epsilon_{0} \rightarrow\right.\) permittivity of free space,
\(h \rightarrow\) planck's constant]
356387
Assuming the atom is in the ground state, the expression for the magnetic field at a point nucleus in hydrogen atom due to circular motion of elecrton is
[ \(\mu_{0} \rightarrow\) permeability of free space,
\(m \rightarrow\) mass of electrons]
\(\left[\epsilon_{0} \rightarrow\right.\) permittivity of free space,
\(h \rightarrow\) planck's constant]
356387
Assuming the atom is in the ground state, the expression for the magnetic field at a point nucleus in hydrogen atom due to circular motion of elecrton is
[ \(\mu_{0} \rightarrow\) permeability of free space,
\(m \rightarrow\) mass of electrons]
\(\left[\epsilon_{0} \rightarrow\right.\) permittivity of free space,
\(h \rightarrow\) planck's constant]