356377
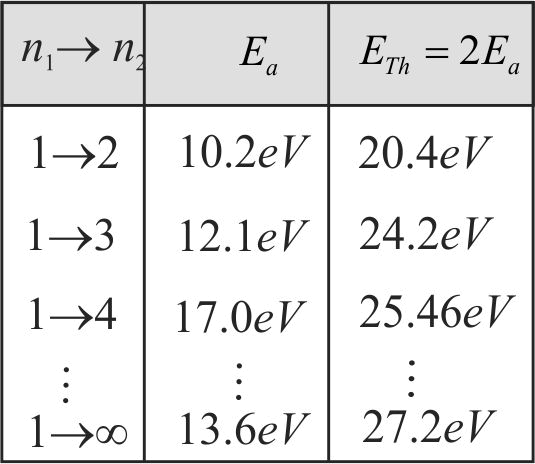
A moving hydrogen atom makes a head-on collision with a stationary hydrogen atom. Before collision, both atoms are in ground state and after collision they move together. What is the minimum value of the kinetic energy of the moving hydrogen atom, such that one of the atoms reaches one of the excitation state?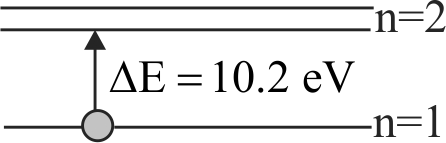
356378 A hydrogen atom moves with a velocity \({u}\) and makes a head on inelastic collision are in the ground state before collision. What is the minimum value of \({u}\), if one of them is to be given a minimum excitation energy? The ionization energy is \(13.6\,eV\). Mass of the hydrogen atom is \({1.0078 \times 1.66 \times 10^{-27} {~kg}}\).
356377
A moving hydrogen atom makes a head-on collision with a stationary hydrogen atom. Before collision, both atoms are in ground state and after collision they move together. What is the minimum value of the kinetic energy of the moving hydrogen atom, such that one of the atoms reaches one of the excitation state?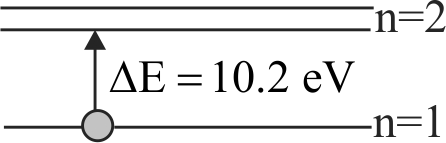
356378 A hydrogen atom moves with a velocity \({u}\) and makes a head on inelastic collision are in the ground state before collision. What is the minimum value of \({u}\), if one of them is to be given a minimum excitation energy? The ionization energy is \(13.6\,eV\). Mass of the hydrogen atom is \({1.0078 \times 1.66 \times 10^{-27} {~kg}}\).
356377
A moving hydrogen atom makes a head-on collision with a stationary hydrogen atom. Before collision, both atoms are in ground state and after collision they move together. What is the minimum value of the kinetic energy of the moving hydrogen atom, such that one of the atoms reaches one of the excitation state?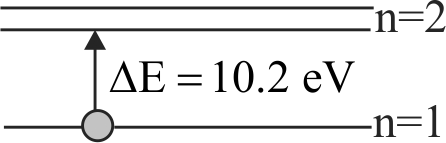
356378 A hydrogen atom moves with a velocity \({u}\) and makes a head on inelastic collision are in the ground state before collision. What is the minimum value of \({u}\), if one of them is to be given a minimum excitation energy? The ionization energy is \(13.6\,eV\). Mass of the hydrogen atom is \({1.0078 \times 1.66 \times 10^{-27} {~kg}}\).
356377
A moving hydrogen atom makes a head-on collision with a stationary hydrogen atom. Before collision, both atoms are in ground state and after collision they move together. What is the minimum value of the kinetic energy of the moving hydrogen atom, such that one of the atoms reaches one of the excitation state?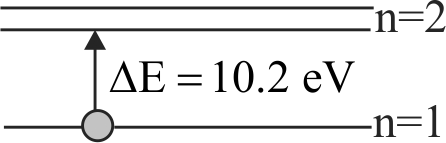
356378 A hydrogen atom moves with a velocity \({u}\) and makes a head on inelastic collision are in the ground state before collision. What is the minimum value of \({u}\), if one of them is to be given a minimum excitation energy? The ionization energy is \(13.6\,eV\). Mass of the hydrogen atom is \({1.0078 \times 1.66 \times 10^{-27} {~kg}}\).
356377
A moving hydrogen atom makes a head-on collision with a stationary hydrogen atom. Before collision, both atoms are in ground state and after collision they move together. What is the minimum value of the kinetic energy of the moving hydrogen atom, such that one of the atoms reaches one of the excitation state?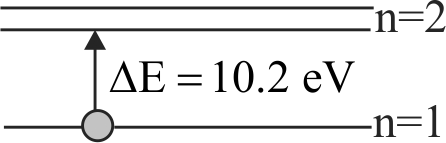
356378 A hydrogen atom moves with a velocity \({u}\) and makes a head on inelastic collision are in the ground state before collision. What is the minimum value of \({u}\), if one of them is to be given a minimum excitation energy? The ionization energy is \(13.6\,eV\). Mass of the hydrogen atom is \({1.0078 \times 1.66 \times 10^{-27} {~kg}}\).