356141
Read the Statement - A and Statement - B carefully to mark the correct options given below:
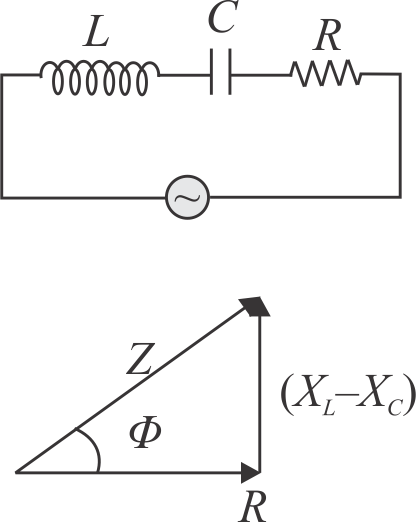
Statement A :
Maximum power is dissipated in a circuit containing an inductor, a capacitor and a resistor connected in series with an \(A C\) source, when resonance occurs.
Statement B :
Maximum power is dissipated in a circuit containing pure resistor due to zero phase difference between current and voltage.
356141
Read the Statement - A and Statement - B carefully to mark the correct options given below:
Statement A :
Maximum power is dissipated in a circuit containing an inductor, a capacitor and a resistor connected in series with an \(A C\) source, when resonance occurs.
Statement B :
Maximum power is dissipated in a circuit containing pure resistor due to zero phase difference between current and voltage.
356141
Read the Statement - A and Statement - B carefully to mark the correct options given below:
Statement A :
Maximum power is dissipated in a circuit containing an inductor, a capacitor and a resistor connected in series with an \(A C\) source, when resonance occurs.
Statement B :
Maximum power is dissipated in a circuit containing pure resistor due to zero phase difference between current and voltage.
356141
Read the Statement - A and Statement - B carefully to mark the correct options given below:
Statement A :
Maximum power is dissipated in a circuit containing an inductor, a capacitor and a resistor connected in series with an \(A C\) source, when resonance occurs.
Statement B :
Maximum power is dissipated in a circuit containing pure resistor due to zero phase difference between current and voltage.
356141
Read the Statement - A and Statement - B carefully to mark the correct options given below:
Statement A :
Maximum power is dissipated in a circuit containing an inductor, a capacitor and a resistor connected in series with an \(A C\) source, when resonance occurs.
Statement B :
Maximum power is dissipated in a circuit containing pure resistor due to zero phase difference between current and voltage.