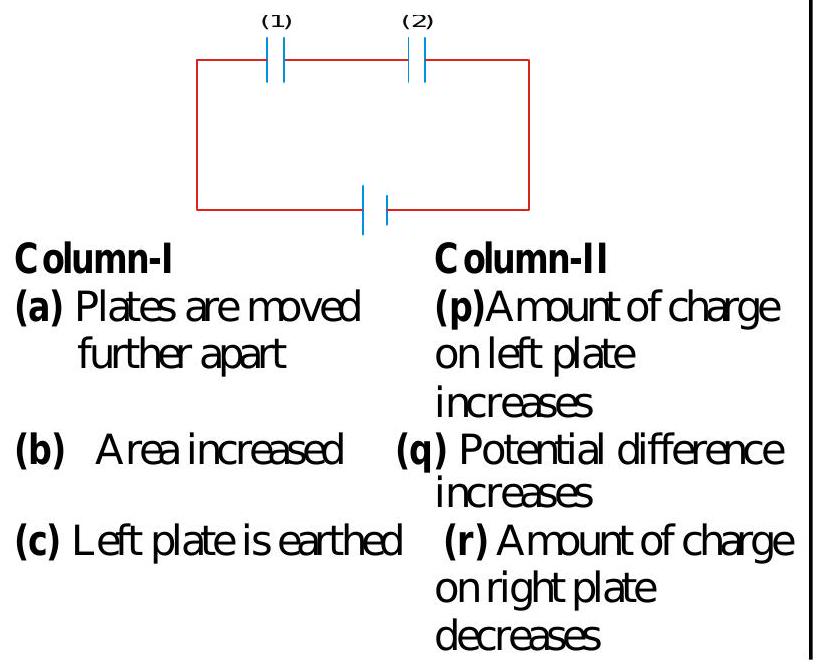
268049
Three identical condensersare connected together in four different ways. First all of them are connected in series and the equivalent capacity is \(\mathrm{C}_{1}\). Next all of them are connected in parallel and the equivalent capacity is \(\mathrm{C}_{2^{2}}\). Next two of them are connected in series and the third one connected in parallel to the combination and the equivalent capacity is \(\mathrm{C}_{3}\). Next two of them are connected in parallel and the third
one connected in series with the combination and the equivalent capacity is \(\mathrm{C}_{4}\). Which of the following is correct ascending order of the equivalent capacities?
268050
On a capacitor of capacitance\(C_{0}\) following steps are performed in the order as given in column I.
(A) Capacitor is charged by connecting it across a battery of emf \(E_{0}\)
(B) Dielectric of dielectric constant \(K\) and thickness d is inserted
(C) C apacitor is disconnected from battery
(D) Separation between plates is doubled
|Column-I (Steps performed) |Column-II (Final value of Q uantity (Symbols have usual meaning) (Steps performed)|
|-----|------|
|(a) \((A)(D)(C)(B)\)|(p) \(Q=\frac{C_{0} E_{0}}{2}\)|
|(b) (D)(A)(C)(B)|(q) \(Q=\frac{K C_{0} E_{0}}{K+1}\)|
|(c) \((B)(A)(C)(D)\)|(r) \(C=\frac{K C_{0}}{K+1}\)|
|(d) \((A)(B)(D)(C)\)|(s) \(V=\frac{E_{0}(K+1)}{2 K}\)|
268052
The potential across a\(3 \mu \mathrm{F}\) capacitor is \(12 \mathrm{~V}\) when it is not connected to anything. It is then connected in parallel with an uncharged \(6 \mu \mathrm{F}\) capacitor. At equilibrium, the charge and potential difference across the capacitor 3 \(\mu \mathrm{F}\) and \(6 \mu \mathrm{F}\) arelisted in column I. M atch it with column III.
|Column-I|Column-II|
|------|------|
|(a) charge on \(3 \mu \mathrm{F}\) capacitor|(p) \(12 \mu \mathrm{C}\)|
|(b) charge on \(6 \mu \mathrm{F}\) capacitor|(q) \(24 \mu \mathrm{F}\)|
|(c) potential difference across \(3 \mu \mathrm{F}\) |(r) \(8 \mathrm{~V}\) capacitor|
|(d) potential difference across \(6 \mu \mathrm{F}\) |(s) \(4 \mathrm{~V}\) capacitor|
268049
Three identical condensersare connected together in four different ways. First all of them are connected in series and the equivalent capacity is \(\mathrm{C}_{1}\). Next all of them are connected in parallel and the equivalent capacity is \(\mathrm{C}_{2^{2}}\). Next two of them are connected in series and the third one connected in parallel to the combination and the equivalent capacity is \(\mathrm{C}_{3}\). Next two of them are connected in parallel and the third
one connected in series with the combination and the equivalent capacity is \(\mathrm{C}_{4}\). Which of the following is correct ascending order of the equivalent capacities?
268050
On a capacitor of capacitance\(C_{0}\) following steps are performed in the order as given in column I.
(A) Capacitor is charged by connecting it across a battery of emf \(E_{0}\)
(B) Dielectric of dielectric constant \(K\) and thickness d is inserted
(C) C apacitor is disconnected from battery
(D) Separation between plates is doubled
|Column-I (Steps performed) |Column-II (Final value of Q uantity (Symbols have usual meaning) (Steps performed)|
|-----|------|
|(a) \((A)(D)(C)(B)\)|(p) \(Q=\frac{C_{0} E_{0}}{2}\)|
|(b) (D)(A)(C)(B)|(q) \(Q=\frac{K C_{0} E_{0}}{K+1}\)|
|(c) \((B)(A)(C)(D)\)|(r) \(C=\frac{K C_{0}}{K+1}\)|
|(d) \((A)(B)(D)(C)\)|(s) \(V=\frac{E_{0}(K+1)}{2 K}\)|
268052
The potential across a\(3 \mu \mathrm{F}\) capacitor is \(12 \mathrm{~V}\) when it is not connected to anything. It is then connected in parallel with an uncharged \(6 \mu \mathrm{F}\) capacitor. At equilibrium, the charge and potential difference across the capacitor 3 \(\mu \mathrm{F}\) and \(6 \mu \mathrm{F}\) arelisted in column I. M atch it with column III.
|Column-I|Column-II|
|------|------|
|(a) charge on \(3 \mu \mathrm{F}\) capacitor|(p) \(12 \mu \mathrm{C}\)|
|(b) charge on \(6 \mu \mathrm{F}\) capacitor|(q) \(24 \mu \mathrm{F}\)|
|(c) potential difference across \(3 \mu \mathrm{F}\) |(r) \(8 \mathrm{~V}\) capacitor|
|(d) potential difference across \(6 \mu \mathrm{F}\) |(s) \(4 \mathrm{~V}\) capacitor|
268049
Three identical condensersare connected together in four different ways. First all of them are connected in series and the equivalent capacity is \(\mathrm{C}_{1}\). Next all of them are connected in parallel and the equivalent capacity is \(\mathrm{C}_{2^{2}}\). Next two of them are connected in series and the third one connected in parallel to the combination and the equivalent capacity is \(\mathrm{C}_{3}\). Next two of them are connected in parallel and the third
one connected in series with the combination and the equivalent capacity is \(\mathrm{C}_{4}\). Which of the following is correct ascending order of the equivalent capacities?
268050
On a capacitor of capacitance\(C_{0}\) following steps are performed in the order as given in column I.
(A) Capacitor is charged by connecting it across a battery of emf \(E_{0}\)
(B) Dielectric of dielectric constant \(K\) and thickness d is inserted
(C) C apacitor is disconnected from battery
(D) Separation between plates is doubled
|Column-I (Steps performed) |Column-II (Final value of Q uantity (Symbols have usual meaning) (Steps performed)|
|-----|------|
|(a) \((A)(D)(C)(B)\)|(p) \(Q=\frac{C_{0} E_{0}}{2}\)|
|(b) (D)(A)(C)(B)|(q) \(Q=\frac{K C_{0} E_{0}}{K+1}\)|
|(c) \((B)(A)(C)(D)\)|(r) \(C=\frac{K C_{0}}{K+1}\)|
|(d) \((A)(B)(D)(C)\)|(s) \(V=\frac{E_{0}(K+1)}{2 K}\)|
268052
The potential across a\(3 \mu \mathrm{F}\) capacitor is \(12 \mathrm{~V}\) when it is not connected to anything. It is then connected in parallel with an uncharged \(6 \mu \mathrm{F}\) capacitor. At equilibrium, the charge and potential difference across the capacitor 3 \(\mu \mathrm{F}\) and \(6 \mu \mathrm{F}\) arelisted in column I. M atch it with column III.
|Column-I|Column-II|
|------|------|
|(a) charge on \(3 \mu \mathrm{F}\) capacitor|(p) \(12 \mu \mathrm{C}\)|
|(b) charge on \(6 \mu \mathrm{F}\) capacitor|(q) \(24 \mu \mathrm{F}\)|
|(c) potential difference across \(3 \mu \mathrm{F}\) |(r) \(8 \mathrm{~V}\) capacitor|
|(d) potential difference across \(6 \mu \mathrm{F}\) |(s) \(4 \mathrm{~V}\) capacitor|
268049
Three identical condensersare connected together in four different ways. First all of them are connected in series and the equivalent capacity is \(\mathrm{C}_{1}\). Next all of them are connected in parallel and the equivalent capacity is \(\mathrm{C}_{2^{2}}\). Next two of them are connected in series and the third one connected in parallel to the combination and the equivalent capacity is \(\mathrm{C}_{3}\). Next two of them are connected in parallel and the third
one connected in series with the combination and the equivalent capacity is \(\mathrm{C}_{4}\). Which of the following is correct ascending order of the equivalent capacities?
268050
On a capacitor of capacitance\(C_{0}\) following steps are performed in the order as given in column I.
(A) Capacitor is charged by connecting it across a battery of emf \(E_{0}\)
(B) Dielectric of dielectric constant \(K\) and thickness d is inserted
(C) C apacitor is disconnected from battery
(D) Separation between plates is doubled
|Column-I (Steps performed) |Column-II (Final value of Q uantity (Symbols have usual meaning) (Steps performed)|
|-----|------|
|(a) \((A)(D)(C)(B)\)|(p) \(Q=\frac{C_{0} E_{0}}{2}\)|
|(b) (D)(A)(C)(B)|(q) \(Q=\frac{K C_{0} E_{0}}{K+1}\)|
|(c) \((B)(A)(C)(D)\)|(r) \(C=\frac{K C_{0}}{K+1}\)|
|(d) \((A)(B)(D)(C)\)|(s) \(V=\frac{E_{0}(K+1)}{2 K}\)|
268052
The potential across a\(3 \mu \mathrm{F}\) capacitor is \(12 \mathrm{~V}\) when it is not connected to anything. It is then connected in parallel with an uncharged \(6 \mu \mathrm{F}\) capacitor. At equilibrium, the charge and potential difference across the capacitor 3 \(\mu \mathrm{F}\) and \(6 \mu \mathrm{F}\) arelisted in column I. M atch it with column III.
|Column-I|Column-II|
|------|------|
|(a) charge on \(3 \mu \mathrm{F}\) capacitor|(p) \(12 \mu \mathrm{C}\)|
|(b) charge on \(6 \mu \mathrm{F}\) capacitor|(q) \(24 \mu \mathrm{F}\)|
|(c) potential difference across \(3 \mu \mathrm{F}\) |(r) \(8 \mathrm{~V}\) capacitor|
|(d) potential difference across \(6 \mu \mathrm{F}\) |(s) \(4 \mathrm{~V}\) capacitor|
268049
Three identical condensersare connected together in four different ways. First all of them are connected in series and the equivalent capacity is \(\mathrm{C}_{1}\). Next all of them are connected in parallel and the equivalent capacity is \(\mathrm{C}_{2^{2}}\). Next two of them are connected in series and the third one connected in parallel to the combination and the equivalent capacity is \(\mathrm{C}_{3}\). Next two of them are connected in parallel and the third
one connected in series with the combination and the equivalent capacity is \(\mathrm{C}_{4}\). Which of the following is correct ascending order of the equivalent capacities?
268050
On a capacitor of capacitance\(C_{0}\) following steps are performed in the order as given in column I.
(A) Capacitor is charged by connecting it across a battery of emf \(E_{0}\)
(B) Dielectric of dielectric constant \(K\) and thickness d is inserted
(C) C apacitor is disconnected from battery
(D) Separation between plates is doubled
|Column-I (Steps performed) |Column-II (Final value of Q uantity (Symbols have usual meaning) (Steps performed)|
|-----|------|
|(a) \((A)(D)(C)(B)\)|(p) \(Q=\frac{C_{0} E_{0}}{2}\)|
|(b) (D)(A)(C)(B)|(q) \(Q=\frac{K C_{0} E_{0}}{K+1}\)|
|(c) \((B)(A)(C)(D)\)|(r) \(C=\frac{K C_{0}}{K+1}\)|
|(d) \((A)(B)(D)(C)\)|(s) \(V=\frac{E_{0}(K+1)}{2 K}\)|
268052
The potential across a\(3 \mu \mathrm{F}\) capacitor is \(12 \mathrm{~V}\) when it is not connected to anything. It is then connected in parallel with an uncharged \(6 \mu \mathrm{F}\) capacitor. At equilibrium, the charge and potential difference across the capacitor 3 \(\mu \mathrm{F}\) and \(6 \mu \mathrm{F}\) arelisted in column I. M atch it with column III.
|Column-I|Column-II|
|------|------|
|(a) charge on \(3 \mu \mathrm{F}\) capacitor|(p) \(12 \mu \mathrm{C}\)|
|(b) charge on \(6 \mu \mathrm{F}\) capacitor|(q) \(24 \mu \mathrm{F}\)|
|(c) potential difference across \(3 \mu \mathrm{F}\) |(r) \(8 \mathrm{~V}\) capacitor|
|(d) potential difference across \(6 \mu \mathrm{F}\) |(s) \(4 \mathrm{~V}\) capacitor|