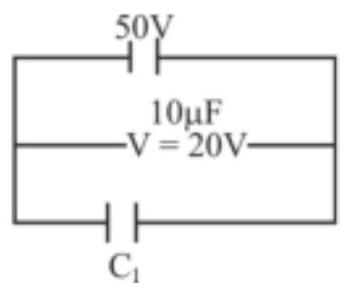
165968 The plates of a parallel plate capacitor are charged upto $200 \mathrm{~V}$. A dielectric slab of thickness $4 \mathrm{~mm}$ is inserted between its plates. Then, to maintain the same potential difference between the plates of the capacitor, the distance between the plates is increased by $3.2 \mathrm{~mm}$. The dielectric constant of the dielectric slab is

165969 A $10 \mu \mathrm{F}$ capacitor is charged to a potential difference of $50 \mathrm{~V}$ and is connected to another uncharged capacitor in parallel. Now the common potential difference becomes $20 \mathrm{~V}$. The capacitance of second capacitor is

165970 The function of a dielectric in a capacitor is

165971 An air filled parallel plate capacitor charged to potential ' $V_{1}$ ' is connected to uncharged parallel plate capacitor having dielectric constant ' $K$ '. The common potential of both is ' $V_{2}$ '. The value of ' $K$ ' will be

165972 The capacitance of a parallel plate capacitor with air as medium is $3 \mu \mathrm{F}$. With the introduction of a dielectric medium between the plates, the capacitance becomes $15 \mu \mathrm{F}$. The permittivity of the medium in SI unit is $\varepsilon_{0}=$ $8.85 \times 10^{-12}$ SI unit

165968 The plates of a parallel plate capacitor are charged upto $200 \mathrm{~V}$. A dielectric slab of thickness $4 \mathrm{~mm}$ is inserted between its plates. Then, to maintain the same potential difference between the plates of the capacitor, the distance between the plates is increased by $3.2 \mathrm{~mm}$. The dielectric constant of the dielectric slab is

165969 A $10 \mu \mathrm{F}$ capacitor is charged to a potential difference of $50 \mathrm{~V}$ and is connected to another uncharged capacitor in parallel. Now the common potential difference becomes $20 \mathrm{~V}$. The capacitance of second capacitor is

165970 The function of a dielectric in a capacitor is

165971 An air filled parallel plate capacitor charged to potential ' $V_{1}$ ' is connected to uncharged parallel plate capacitor having dielectric constant ' $K$ '. The common potential of both is ' $V_{2}$ '. The value of ' $K$ ' will be

165972 The capacitance of a parallel plate capacitor with air as medium is $3 \mu \mathrm{F}$. With the introduction of a dielectric medium between the plates, the capacitance becomes $15 \mu \mathrm{F}$. The permittivity of the medium in SI unit is $\varepsilon_{0}=$ $8.85 \times 10^{-12}$ SI unit

165968 The plates of a parallel plate capacitor are charged upto $200 \mathrm{~V}$. A dielectric slab of thickness $4 \mathrm{~mm}$ is inserted between its plates. Then, to maintain the same potential difference between the plates of the capacitor, the distance between the plates is increased by $3.2 \mathrm{~mm}$. The dielectric constant of the dielectric slab is

165969 A $10 \mu \mathrm{F}$ capacitor is charged to a potential difference of $50 \mathrm{~V}$ and is connected to another uncharged capacitor in parallel. Now the common potential difference becomes $20 \mathrm{~V}$. The capacitance of second capacitor is

165970 The function of a dielectric in a capacitor is

165971 An air filled parallel plate capacitor charged to potential ' $V_{1}$ ' is connected to uncharged parallel plate capacitor having dielectric constant ' $K$ '. The common potential of both is ' $V_{2}$ '. The value of ' $K$ ' will be

165972 The capacitance of a parallel plate capacitor with air as medium is $3 \mu \mathrm{F}$. With the introduction of a dielectric medium between the plates, the capacitance becomes $15 \mu \mathrm{F}$. The permittivity of the medium in SI unit is $\varepsilon_{0}=$ $8.85 \times 10^{-12}$ SI unit

165968 The plates of a parallel plate capacitor are charged upto $200 \mathrm{~V}$. A dielectric slab of thickness $4 \mathrm{~mm}$ is inserted between its plates. Then, to maintain the same potential difference between the plates of the capacitor, the distance between the plates is increased by $3.2 \mathrm{~mm}$. The dielectric constant of the dielectric slab is

165969 A $10 \mu \mathrm{F}$ capacitor is charged to a potential difference of $50 \mathrm{~V}$ and is connected to another uncharged capacitor in parallel. Now the common potential difference becomes $20 \mathrm{~V}$. The capacitance of second capacitor is

165970 The function of a dielectric in a capacitor is

165971 An air filled parallel plate capacitor charged to potential ' $V_{1}$ ' is connected to uncharged parallel plate capacitor having dielectric constant ' $K$ '. The common potential of both is ' $V_{2}$ '. The value of ' $K$ ' will be

165972 The capacitance of a parallel plate capacitor with air as medium is $3 \mu \mathrm{F}$. With the introduction of a dielectric medium between the plates, the capacitance becomes $15 \mu \mathrm{F}$. The permittivity of the medium in SI unit is $\varepsilon_{0}=$ $8.85 \times 10^{-12}$ SI unit

165968 The plates of a parallel plate capacitor are charged upto $200 \mathrm{~V}$. A dielectric slab of thickness $4 \mathrm{~mm}$ is inserted between its plates. Then, to maintain the same potential difference between the plates of the capacitor, the distance between the plates is increased by $3.2 \mathrm{~mm}$. The dielectric constant of the dielectric slab is

165969 A $10 \mu \mathrm{F}$ capacitor is charged to a potential difference of $50 \mathrm{~V}$ and is connected to another uncharged capacitor in parallel. Now the common potential difference becomes $20 \mathrm{~V}$. The capacitance of second capacitor is

165970 The function of a dielectric in a capacitor is

165971 An air filled parallel plate capacitor charged to potential ' $V_{1}$ ' is connected to uncharged parallel plate capacitor having dielectric constant ' $K$ '. The common potential of both is ' $V_{2}$ '. The value of ' $K$ ' will be

165972 The capacitance of a parallel plate capacitor with air as medium is $3 \mu \mathrm{F}$. With the introduction of a dielectric medium between the plates, the capacitance becomes $15 \mu \mathrm{F}$. The permittivity of the medium in SI unit is $\varepsilon_{0}=$ $8.85 \times 10^{-12}$ SI unit