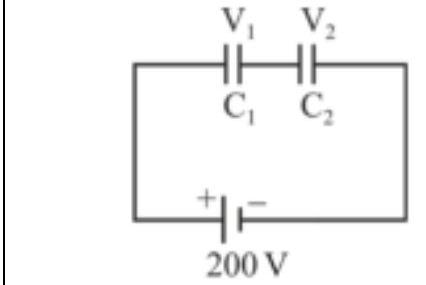
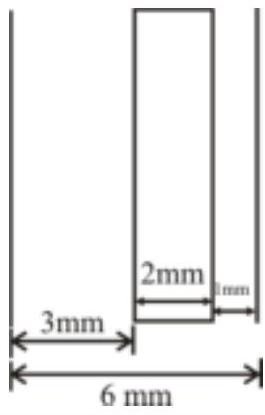
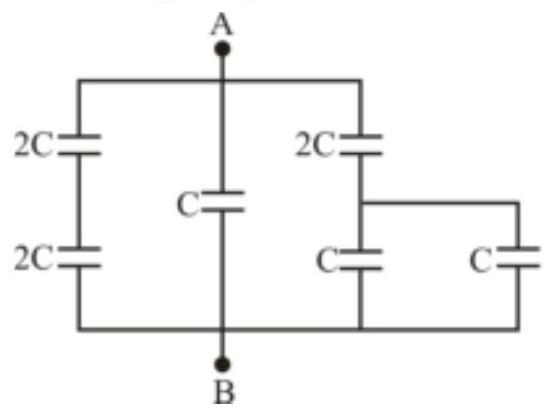
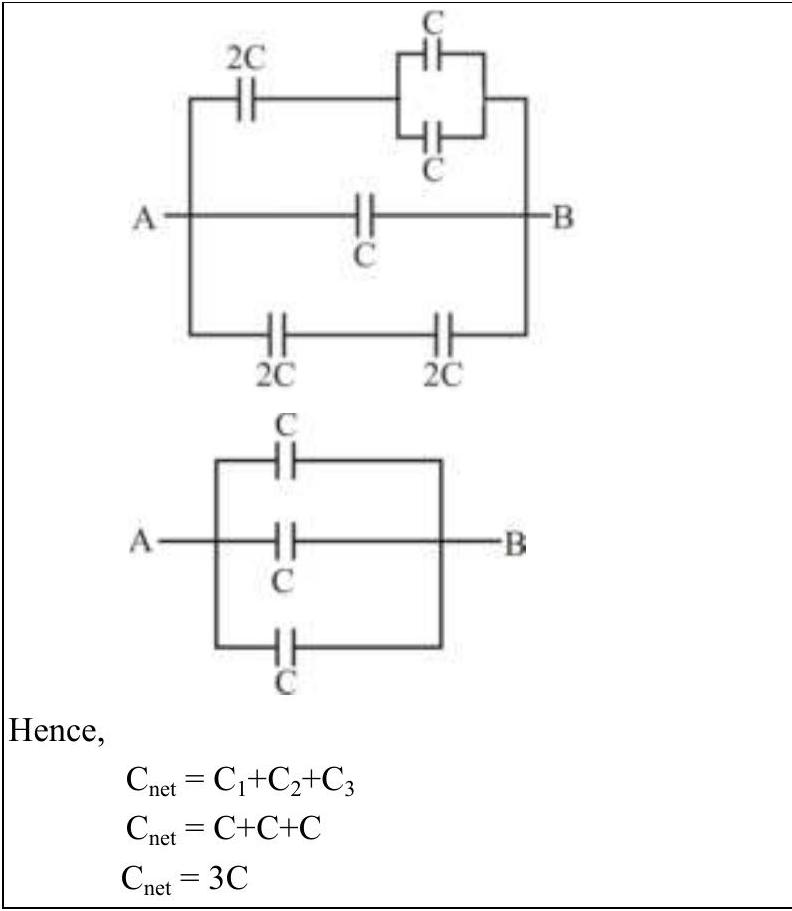
165830 A metal plate of thickness $2 \mathrm{~mm}$ and area $36 \pi$ $\mathbf{c m}^{2}$ - is slide into a parallel plate capacitor of plate spacing $6 \mathrm{~mm}$ and area $36 \pi \mathrm{cm}^{2}$. The metal plate is at a distance $3 \mathrm{~mm}$ from one of the plates. What is the capacitance of this arrangement? $\left(\right.$ Let $\left.\frac{1}{4 \pi \varepsilon_{0}}=9 \times 10^{9} \mathrm{Nm}^{2} \mathrm{C}^{-2}\right)$
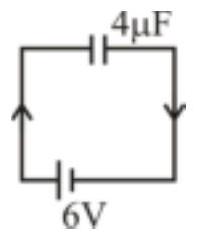
165832 A capacitor of capacitance $4 \mu \mathrm{F}$ is charged to a potential difference of $6 \mathrm{~V}$ with a battery. The battery is removed and in its place another capacitor of capacitance is $8 \mu \mathrm{F}$ introduced and the circuit is closed. The potential difference attained by each of the capacitors in $\mathrm{V}$ is
165830 A metal plate of thickness $2 \mathrm{~mm}$ and area $36 \pi$ $\mathbf{c m}^{2}$ - is slide into a parallel plate capacitor of plate spacing $6 \mathrm{~mm}$ and area $36 \pi \mathrm{cm}^{2}$. The metal plate is at a distance $3 \mathrm{~mm}$ from one of the plates. What is the capacitance of this arrangement? $\left(\right.$ Let $\left.\frac{1}{4 \pi \varepsilon_{0}}=9 \times 10^{9} \mathrm{Nm}^{2} \mathrm{C}^{-2}\right)$
165832 A capacitor of capacitance $4 \mu \mathrm{F}$ is charged to a potential difference of $6 \mathrm{~V}$ with a battery. The battery is removed and in its place another capacitor of capacitance is $8 \mu \mathrm{F}$ introduced and the circuit is closed. The potential difference attained by each of the capacitors in $\mathrm{V}$ is
165830 A metal plate of thickness $2 \mathrm{~mm}$ and area $36 \pi$ $\mathbf{c m}^{2}$ - is slide into a parallel plate capacitor of plate spacing $6 \mathrm{~mm}$ and area $36 \pi \mathrm{cm}^{2}$. The metal plate is at a distance $3 \mathrm{~mm}$ from one of the plates. What is the capacitance of this arrangement? $\left(\right.$ Let $\left.\frac{1}{4 \pi \varepsilon_{0}}=9 \times 10^{9} \mathrm{Nm}^{2} \mathrm{C}^{-2}\right)$
165832 A capacitor of capacitance $4 \mu \mathrm{F}$ is charged to a potential difference of $6 \mathrm{~V}$ with a battery. The battery is removed and in its place another capacitor of capacitance is $8 \mu \mathrm{F}$ introduced and the circuit is closed. The potential difference attained by each of the capacitors in $\mathrm{V}$ is
165830 A metal plate of thickness $2 \mathrm{~mm}$ and area $36 \pi$ $\mathbf{c m}^{2}$ - is slide into a parallel plate capacitor of plate spacing $6 \mathrm{~mm}$ and area $36 \pi \mathrm{cm}^{2}$. The metal plate is at a distance $3 \mathrm{~mm}$ from one of the plates. What is the capacitance of this arrangement? $\left(\right.$ Let $\left.\frac{1}{4 \pi \varepsilon_{0}}=9 \times 10^{9} \mathrm{Nm}^{2} \mathrm{C}^{-2}\right)$
165832 A capacitor of capacitance $4 \mu \mathrm{F}$ is charged to a potential difference of $6 \mathrm{~V}$ with a battery. The battery is removed and in its place another capacitor of capacitance is $8 \mu \mathrm{F}$ introduced and the circuit is closed. The potential difference attained by each of the capacitors in $\mathrm{V}$ is